The orbital arrangement of an atom's electrons. Negatively charged electrons are attracted to a positively charged nucleus to form an atom or ion. Although such bound electrons exhibit a high degree of quantum-mechanical wavelike behavior, there still remain particle aspects to their motion. Bound electrons occupy orbitals that are somewhat concentrated in spatial shells lying at different distances from the nucleus. As the set of electron energies allowed by quantum mechanics is discrete, so is the set of mean shell radii. Both these quantized physical quantities are primarily specified by integral values of the principal, or total, quantum number n. The full electron configuration of an atom is correlated with a set of values for all the quantum numbers of each and every electron. In addition to n, another important quantum number is l, an integer representing the orbital angular momentum of an electron in units of h/2π where h is Planck's constant. The values 1, 2, 3, 4, 5, 6, 7 for n and 0, 1, 2, 3, for l together suffice to describe the electron configurations of all known normal atoms and ions, that is, those that have their lowest possible values of total electronic energy. The first seven shells are also given the letter designations K, L, M, N, O, P, and Q, respectively. Electrons with l equal to 0, 1, 2, and 3 are designated s, p, d, and f, respectively. See also: Quantum mechanics; Quantum numbers
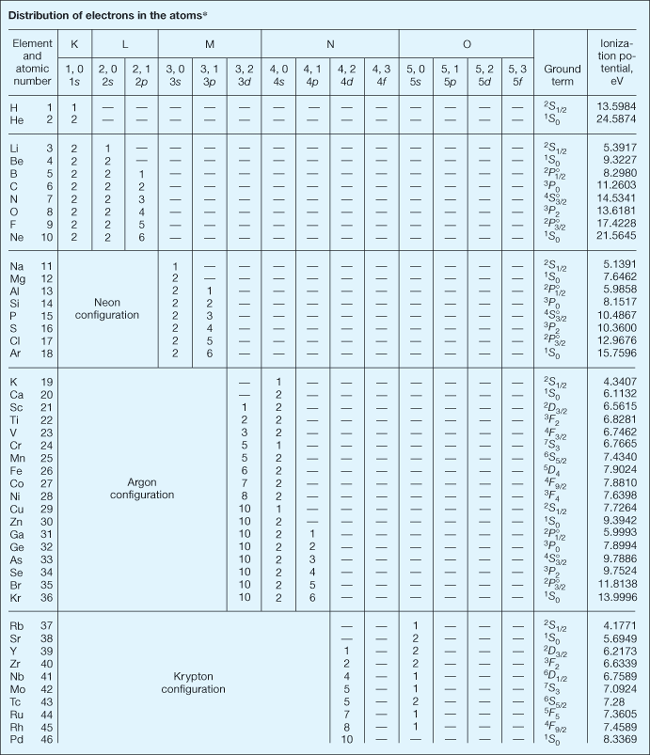
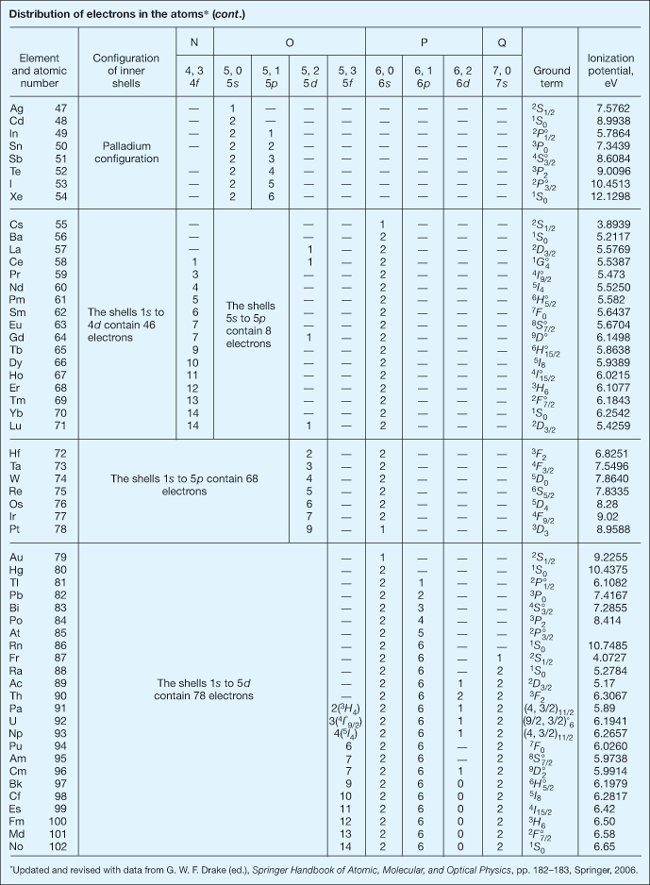
In any configuration the number of equivalent electrons (same n and l) is indicated by an integral exponent (not a quantum number) attached to the letters s, p, d, and f. According to the Pauli exclusion principle the maximum is s2, p6, d10, and f14. For example, the configuration 1s22s22p63s of the ground or lowest energy state of sodium means that there are two electrons with n = 1, l = 0; two with n = 2, l = 0; six with n = 2, l = 1; and one with n = 3, l = 0. Higher values of n and l can be achieved by excitation of normal atoms, either through photon absorption or by particle impact, temporarily moving one or more electrons to unfilled shells of larger radii and increasing the atom's electronic energy. Such excited electronic configurations are inherently unstable. Excited electrons ultimately fall back down to their normal locations closer to the nucleus, a process accompanied by the emission of one or more quanta of electromagnetic radiation (photons). While the atom is excited, the partially depleted shells are said to possess vacancies. See also: Exclusion principle
An electron configuration is categorized as having even or odd parity, according to whether the sum of p and f electrons is even or odd. Strong spectral lines result only from transitions between configurations of unlike parity. See also: Parity (quantum mechanics)
Insofar as they are known from spectroscopic investigations, the electron configurations characteristic of the normal or ground states of the first 102 chemical elements are shown in the table. In the next-to-last column of the table, the spectral term of the energy level with lowest total electronic energy is shown. For all elements except protactinium (Pa), uranium (U), and neptunium (Np), an LS-coupling term is given. Here, the main part of the term symbol is a capital letter, S, P, D, F, and so on, that represents the total electronic orbital angular momentum. Attached to this is a superior prefix, 1, 2, 3, 4, and so on, that indicates the multiplicity, and an anterior suffix, 0, ½, 1, 3/2, 2, 5/2, and so on, that shows the total angular momentum, or J value, of the atom in the given state. The sign ° above the J value signifies that the spectral term and electron configuration have odd parity. (For the elements Pa, U, and Np, a jj coupling term is given. Here, parentheses enclose two numbers, the first of which is the total angular momentum of the electrons in the 5f shell, while the second is the total angular momentum of the electron in the 6d shell. The suffixes following the parentheses are the same as in LS coupling. For these elements the LS coupling of the electrons in the 5f shell is also given in the column that lists the number of such electrons.)
The last column of the table presents the first ionization potential of the atom when this has been derived from spectroscopic observations. In any atomic spectrum, two or more spectral lines with certain similar properties may form a series such that the reciprocal wavelengths 1/λ (number of waves per centimeter = σ) can be closely represented by a formula of the Rydberg type, σ = L − R/(n + μ)2, in which L is the limit of the series. R is called the Rydberg constant, and the principal quantum number n has successive integral values to which a constant fractional part μ is added. The second term vanishes when n approaches infinity, and the series limit is thus evaluated. This limit is usually coincident with the ground state of the ion, and is thus a measure (in wave-number units) of the energy required to remove from an atom its least firmly bound electron and transform a neutral atom into a singly charged ion. The energy required to ionize an atom is usually expressed in electronvolts (1 eV = 8065.48 wave numbers) and is called its first ionization potential. See also: Atomic structure and spectra; Ionization potential; Rydberg atom; Rydberg constant
Molecules consist of two or more atoms that at least partially share one or more electrons with one another. Sets of quantum numbers specify molecular electron configurations in much the same way as for atoms. However, the physical meaning of some molecular quantum numbers is different than that for the atomic case, as is the term notation. See also: Molecular structure and spectra





