Changes of any property of an aggregation of matter and energy, accompanied by thermal effects. The participants in a process are first identified as a system to be studied; the boundaries of the system are established; the initial state of the system is determined; the path of the changing states is laid out; and, finally, supplementary data are stated to establish the thermodynamic process. These steps will be explained in the following paragraphs. At all times it must be remembered that the only processes which are allowed are those compatible with the first and second laws of thermodynamics: Energy is neither created nor destroyed and the entropy of the system plus its surroundings always increases.
A system and its boundaries
To evaluate the results of a process, it is necessary to know the participants that undergo the process, and their mass and energy. A region, or a system, is selected for study, and its contents determined. This region may have both mass and energy entering or leaving during a particular change of conditions, and these mass and energy transfers may result in changes both within the system and within the surroundings which envelop the system.
As the system undergoes a particular change of condition, such as a balloon collapsing due to the escape of gas or a liquid solution brought to a boil in a nuclear reactor, the transfers of mass and energy which occur can be evaluated at the boundaries of the arbitrarily defined system under analysis.
A question that immediately arises is whether a system such as a tank of compressed air should have boundaries which include or exclude the metal walls of the tank. The answer depends upon the aim of the analysis. If its aim is to establish a relationship among the physical properties of the gas, such as to determine how the pressure of the gas varies with the gas temperature at a constant volume, then only the behavior of the gas is involved; the metal walls do not belong within the system. However, if the problem is to determine how much externally applied heat would be required to raise the temperature of the enclosed gas a given amount, then the specific heat of the metal walls, as well as that of the gas, must be considered, and the system boundaries should include the walls through which the heat flows to reach the gaseous contents. In the laboratory, regardless of where the system boundaries are taken, the walls will always play a role and must be reckoned with.
State of a system
To establish the exact path of a process, the initial state of the system must be determined, specifying the values of variables such as temperature, pressure, volume, and quantity of material. If a number of chemicals are present in the system, the number of variables needed is usually equal to the number of independently variable substances present plus two such as temperature and pressure; exceptions to this rule occur in variable electric or magnetic fields and in some other well-defined cases. Thus, the number of properties required to specify the state of a system depends upon the complexity of the system. Whenever a system changes from one state to another, a process occurs.
Whenever an unbalance occurs in an intensive property such as temperature, pressure, or density, either within the system or between the system and its surroundings, the force of the unbalance can initiate a process that causes a change of state. Examples are the unequal molecular concentration of different gases within a single rigid enclosure, a difference of temperature across the system boundary, a difference of pressure normal to a nonrigid system boundary, or a difference of electrical potential across an electrically conducting system boundary. The direction of the change of state caused by the unbalanced force is such as to reduce the unbalanced driving potential. Rates of changes of state tend to decelerate as this driving potential is decreased.
Equilibrium
The decelerating rate of change implies that all states move toward new conditions of equilibrium. When there are no longer any balanced forces acting within the boundaries of a system or between the system and its surroundings, then no mechanical changes can take place, and the system is said to be in mechanical equilibrium. A system in mechanical equilibrium, such as a mixture of hydrogen and oxygen, under certain conditions might undergo a chemical change. However, if there is no net change in the chemical constituents, then the mixture is said to be in chemical as well as in mechanical equilibrium.
If all parts of a system in chemical and mechanical equilibrium attain a uniform temperature and if, in addition, the system and its surroundings either are at the same temperature or are separated by a thermally nonconducting boundary, then the system has also reached a condition of thermal equilibrium.
Whenever a system is in mechanical, chemical, and thermal equilibrium, so that no mechanical, chemical, or thermal changes can occur, the system is in thermodynamic equilibrium. The state of equilibrium is at a point where the tendency of the system to minimize its energy is balanced by the tendency toward a condition of maximum randomness. In thermodynamics, the state of a system can be defined only when it is in equilibrium. The static state on a macroscopic level is nevertheless underlaid by rapid molecular changes; thermodynamic equilibrium is a condition where the forward and reverse rates of the various changes are all equal to one another. In general, those systems considered in thermodynamics can include not only mixtures of material substances but also mixtures of matter and all forms of energy. For example, one could consider the equilibrium between a gas of charged particles and electromagnetic radiation contained in an oven.
Process path
If under the influence of an unbalanced intensive factor the state of a system is altered, then the change of state of the system is described in terms of the end states or difference between the initial and final properties.
The path of a change of state is the locus of the whole series of states through which the system passes when going from an initial to a final state. For example, suppose a gas expands to twice its volume and that its initial and final temperatures are the same. Various paths connect these initial and final states: isothermal expansion, with temperature held constant at all times, or adiabatic expansion which results in cooling followed by heating back to the initial temperature while holding volume fixed.
Each of these paths can be altered by making the gas do varying amounts of work by pushing out a piston during the expansion, so that an extremely large number of paths can be followed even for such a simple example. The detailed path must be specified if the heat or work is to be a known quantity; however, changes in the thermodynamic properties depend only on the initial and final states and not upon the path.
There are several corollaries from the above descriptions of systems, boundaries, states, and processes. First, all thermodynamic properties are identical for identical states. Second, the change in a property between initial and final states is independent of path or processes. The third corollary is that a quantity whose change is fixed by the end states and is independent of the path is a point function or a property. However, it must be remembered that by the second law of thermodynamics not all states are available (possible final states) from a given initial state and not all conceivable paths are possible in going toward an available state.
Pressure-volume-temperature diagram
Whereas the state of a system is a point function, the change of state of a system, or a process, is a path function. Various processes or methods of change of a system from one state to another may be depicted graphically as a path on a plot using thermodynamic properties as coordinates.
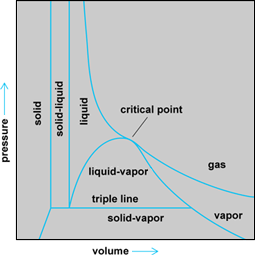
The variable properties most frequently and conveniently measured are pressure, volume, and temperature. If any two of these are held fixed (independent variables), the third is determined (dependent variable). To depict the relationship among these physical properties of the particular working substance, these three variables may be used as the coordinates of a three-dimensional space. The resulting surface is a graphic presentation of the equation of state for this working substance, and all possible equilibrium states of the substance lie on this P-V-T surface. The P-V-T surface may be extensive enough to include all three phases of the working substance: solid, liquid, and vapor.
Because a P-V-T surface represents all equilibrium conditions of the working substance, any line on the surface represents a possible reversible process, or a succession of equilibrium states.
The portion of the P-V-T surface shown in Fig. 1 typifies most real substances; it is characterized by contraction of the substance on freezing. Going from the liquid surface to the liquid-solid surface onto the solid surface involves a decrease in both temperature and volume. Water is one of the few exceptions to this condition; it expands upon freezing, and its resultant P-V-T surface is somewhat modified where the solid and liquid phases abut.

Gibbs' phase rule is defined in Eq. (1).
Here f is the degree of freedom; this integer states the number of intensive properties (such as temperature, pressure, and mole fractions or chemical potentials of the components) which can be varied independently of each other and thereby fix the particular equilibrium state of the system (see discussion under “Temperature-entropy diagram” below). Also, p indicates the number of phases (gas, liquid, or solid) and c the number of component substances in the system. Consider a one-component system (a pure substance) which is either in the liquid, gaseous, or solid phase. In equilibrium the system has two degrees of freedom; that is, two independent thermodynamic properties must be chosen to specify the state. Among the thermodynamic properties of a substance which can be quantitatively evaluated are the pressure, temperature, specific volume, internal energy, enthalpy, and entropy. From among these properties, any two may be selected. If these two prove to be independent of each other, when the values of these two properties are fixed, the state is determined and the values of all the other properties are also fixed. A one-component system with two phases in equilibrium (such as liquid in equilibrium with its vapor in a closed vessel) has f = 1; that is, only one intensive property can be independently specified. Also, a one-component system with three phases in equilibrium has no degree of freedom. Examination of Fig. 1 shows that the three surfaces (solid-liquid, solid-vapor, and liquid-vapor) are generated by lines parallel to the volume axis. Moving the system along such lines (constant pressure and temperature) involves a heat exchange and a change in the relative proportion of the two phases. Note that there is an entropy increment associated with this change.
One can project the three-dimensional surface onto the P-T plane as in Fig. 2. The triple point is the point where the three phases are in equilibrium. When the temperature exceeds the critical temperature (at the critical point), only the gaseous phase is possible. The gas is called a vapor when it can coexist with another phase (at temperatures below the critical point). The P-T diagram for water would have the solid-liquid curve going upward from the triple point to the left (contrary to the ordinary substance pictured in Fig. 2). Then the property so well known to ice skaters would be evident. As the solid-liquid line is crossed from the low-pressure side to the high-pressure side, the water changes from solid to liquid: ice melts upon application of pressure.

Work of a process
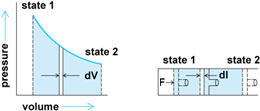
The three-dimensional surface can also be projected onto the P-V plane to get Fig. 3. This plot has a special significance. The area under any reversible path on this plane represents the work done during the process. The fact that this P-V area represents useful work can be demonstrated by the following example.
Let a gas undergo an infinitesimal expansion in a cylinder equipped with a frictionless piston, and let this expansion perform useful work on the surroundings. The work done during this infinitesimal expansion is the force multiplied by the distance through which it acts, as in Eq. (2),
wherein dW is an infinitesimally small work quantity, F is the force, and dl is the infinitesimal distance through which F acts.
But force F is equal to the pressure P of the fluid times the area A of the piston, or PA. However, the product of the area of the piston times the infinitesimal displacement is really the infinitesimal volume swept by the piston, or A dl = dV, with dV equal to an infinitesimal volume. Thus Eq. (3)
is valid. The work term is found by integration, as in Eq. (4).
Figure 4 shows that the integral represents the area under the path described by the expansion from state 1 to state 2 on the P-V plane. Thus, the area on the P-V plane represents work done during this expansion process.
Temperature-entropy diagram
Energy quantities may be depicted as the product of two factors: an intensive property and an extensive one. Examples of intensive properties are pressure, temperature, and magnetic field; extensive ones are volume, magnetization, and mass. Thus, in differential form, work has been presented as the product of a pressure exerted against an area which sweeps through an infinitesimal volume, as in Eq. (5).
Note that as a gas expands, it is doing work on its environment. However, a number of different kinds of work are known. For example, one could have work on polarization of a dielectric, of magnetization, of stretching a wire, or of making new surface area. In all cases, the infinitesimal work is given by Eq. (6),
where X is a generalized applied force which is an intensive quantity such as voltage, magnetic field, or surface tension; and dx is a generalized displacement of the system and is thus extensive. Examples of dx include changes in electric polarization, magnetization, length of a stretched wire, or surface area.
By extending this approach, one can depict transferred heat as the product of an intensive property, temperature, and a distributed or extensive property, defined as entropy, for which the symbol is S. See also: Entropy
If an infinitesimal quantity of heat dQ is transferred during a reversible process, this process may be expressed mathematically as in Eq. (7),
with T being the absolute temperature and dS the infinitesimal entropy quantity.
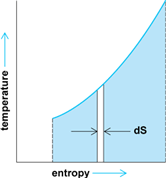
Furthermore, a plot of the change of state of the system undergoing this reversible heat transfer can be drawn on a plane in which the coordinates are absolute temperature and entropy (Fig. 5). The total heat transferred during this process equals the area between this plotted line and the horizontal axis.
Reversible processes
Not all energy contained in or associated with a mass can be converted into useful work. Under ideal conditions only a fraction of the total energy present can be converted into work. The ideal conversions which retain the maximum available useful energy are reversible processes.
Characteristics of a reversible process are that the working substance is always in thermodynamic equilibrium and the process involves no dissipative effects such as viscosity, friction, inelasticity, electrical resistance, or magnetic hysteresis. Thus, reversible processes proceed quasistatically so that the system passes through a series of states of thermodynamic equilibrium, both internally and with its surroundings. This series of states may be traversed just as well in one direction as in the other.
If there are no dissipative effects, all useful work done by the system during a process in one direction can be returned to the system during the reverse process. When such a process is reversed so that the system returns to its starting state, it must leave an effect on the surroundings since, by the second law of thermodynamics, in energy conversion processes the form of energy is always degraded. Part of the energy of the system (including heat source) is transferred as heat from a higher temperature to a lower temperature. The energy rejected to a lower-temperature heat sink cannot be recovered. To return the system (including heat source and sink) to its original state, then, requires more energy than the useful work done by the system during a process in one direction. Of course, if the process were purely a mechanical one with no thermal effects, then both the surroundings and system could be returned to their initial states. See also: Carnot cycle; Thermodynamic cycle
It is impossible to satisfy the conditions of a quasistatic process with no dissipative effects; a reversible process is an ideal abstraction which is not realizable in practice but is useful for theoretical calculations. An ideal reversible engine operating between hotter and cooler bodies at the temperatures T1 and T2, respectively, can put out of the transferred heat energy as useful work.
There are four reversible processes wherein one of the common thermodynamic parameters is kept constant. The general reversible process for a closed or nonflow system is described as a polytropic process. See also: Isentropic process; Isobaric process; Isometric process; Isothermal process; Polytropic process
Irreversible processes
Actual changes of a system deviate from the idealized situation of a quasistatic process devoid of dissipative effects. The extent of the deviation from ideality is correspondingly the extent of the irreversibility of the process.
Real expansions take place in finite time, not infinitely slowly, and these expansions occur with friction of rubbing parts, turbulence of the fluid, pressure waves sweeping across and rebounding through the cylinder, and finite temperature gradients driving the transferred heat. These dissipative effects, the kind of effects that make a pendulum or yo-yo slow down and stop, also make the work output of actual irreversible expansions less than the maximum ideal work of a corresponding reversible process. For a reversible process, as stated earlier, the entropy change is given by . For an irreversible process even more entropy is produced (turbulence and loss of information) and there is the inequality .





