Liquid-vapor equilibrium

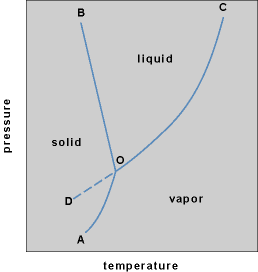
The relationship between the vapor pressure of a liquid and the temperature is indicated by a phase diagram. The illustration shows the phase diagram for water, the line OC being the vapor pressure line for liquid water. Line AO is the vapor pressure line (sublimation pressure curve) for ice, and line BO is the liquid-solid equilibrium line. Point O is called the triple point and is the unique pressure and temperature at which a pure solid, its liquid, and its vapor can coexist in equilibrium under the pressure of the vapor alone. The triple point of water is not the familiar melting point (0°C or 32°F) but rather 0.01°C (32.018°F). The distinction is that the total pressure at the triple point is 4.58 mm (611 pascals), the common vapor pressure of solid and liquid water, whereas the total pressure at the melting point is ordinarily 1 atm (100 kilopascals). Point C is called the critical point and is the point above which there is no distinction between the liquid and gaseous phases. It is not possible to liquefy a gas at temperatures above the critical temperature, regardless of the applied pressure. Necessarily, the surface tension and the latent heat of vaporization become zero at the critical point. For ordinary liquids, the vapor pressure at the critical point is usually about 50 atm (5000 kPa). There is no evidence for a critical point on the solid-liquid equilibrium line, and B is meant to indicate a direction, rather than a point; other phases may appear above B, of course, and alter the direction of the line. It is possible to undercool a liquid below its triple point if crystallization nuclei are absent. The vapor pressure of the undercooled liquid is given by the broken line DO and lies above the equilibrium vapor pressure of the solid. The undercooled liquid is therefore metastable, because the system tends to assume its lowest vapor pressure at equilibrium. See also: Critical phenomena; Triple point

Phase diagram of the water system.

Quantitative relations

For most liquids the relationship between the vapor pressure and temperature can be expressed by an equation having the form of (1), where a, b, and c are constants. The simpler equation (2), where m and n are

(1)

(2)

constants, is often adequate. The change in the vapor pressure of a liquid with temperature may be expressed by the Clausius-Clapeyron equation (3), where

(3)

L_v is the molar latent heat of vaporization and ΔV is the difference in molar volumes of the vapor and liquid at the temperature T. Because the molar volume of the liquid is negligible by comparison with that of the vapor except near the critical point, the Clausius-Clapeyron equation may be written as (4),

(4)

where V is the molar volume of the gas. If the gas follows the ideal gas law (PV = RT), the equation may be further written as (5),

(5)

where R is the gas constant. For moderate ranges of temperature, the latent heat of vaporization is constant, and the equation may be rearranged and integrated to give the useful form of (6).

(6)

From the latent heat of vaporization and the vapor pressure at one temperature, the vapor pressures at other temperatures may thus be calculated.

The vapor pressure of a liquid is lowered when a substance is dissolved in it. At low solute concentrations in nonideal solutions, or at all solute concentrations in ideal solutions, the partial vapor pressure of the liquid in a solution is proportional to the mole fraction of the solvent, Eq. (7),

(7)

where X₁ is the solvent mole fraction and P${}^{\text{0}}{}_{\text{1}}$, is the vapor pressure of the pure solvent. Raoult's law, as this important relationship is known, may also be expressed in the form of Eq. (8),

(8)

where X₂ is the mole fraction of the solute. The lowering of the vapor pressure of the solvent is proportional to the mole fraction of the solute. This relation provides the basis for the determination of the molecular weights of dissolved substances. See also: Concentration scales

Vapor pressure measurement

Because the vapor pressures of liquids range widely, a number of methods have been devised for their measurement. The static method consists of introducing an excess of the liquid into an evacuated system at a given temperature, and measuring the vapor pressure with an attached mercury manometer. It is useful for vapor pressures ranging from a few millimeters of mercury up to several atmospheres. The dynamic method consists of introducing the liquid into a system in which the pressure may be varied, and noting the temperature at which the liquid boils at a given pressure. It is useful for moderately high vapor pressures. The transpiration method consists of bubbling a known volume of an inert gas at a definite pressure through the liquid. The quantity of liquid transported into the carrier gas is determined, and the vapor pressure of the liquid is calculated from relation (9), where P_C is the

(9)

carrier gas pressure and n_L and n_C are the numbers of moles of liquid vaporized and carrier gas, respectively. For the measurement of very low vapor pressures, the Knudsen effusion method may be used. This method is based upon the measurement of the mass of vapor which escapes through a very small hole in a vessel containing a liquid in equilibrium with its vapor. If the diameter of the hole is small compared with the mean free path of the gas molecules, the latter suffer no collisions in their passage through the hole. Equation (10)

(10)

relates the mass of gas which passes per square centimeter per second through the hole and the vapor pressure, where m is the mass of gas per square centimeter per second and M is the molecular weight. The Knudsen method has been used with radioactive isotopes or with mass spectrometers to determine very low vapor pressures. See also: Phase equilibrium

Norman H. Nachtrieb