An estimate of the age of the planet Earth based on the lead isotope systematics. The first meaningful estimate was calculated from the average isotopic composition of terrestrial lead (Pb) available at the surface of the planet. The feasibility of the calculation stems principally from the unique circumstance that two isotopes of the element uranium (235U and 238U) decay to two daughters that are also isotopes of another element, lead (207Pb and 206Pb, respectively). This eliminates the requirement of determining the planetary budget of either of the two elements. The total number of 206Pb and 207Pb atoms are related to their respective parents by the equation of radioactive decay, expressed as Eq. (1) for lead-206 and Eq. (2)
for lead-207, where 238U and 235U are the present planetary content of the two isotopes whose decay constants are λ and λ′ respectively, T is the age of the planet, and subscript p denotes primordial Pb, which existed in the solar nebula prior to the formation of planets. See also: Lead isotopes (geochemistry)
Of the four lead isotopes, 208Pb, 207Pb, 206Pb, and 204Pb, the last is not produced in any known process of natural radioactive decay, and is used as an index isotope. For example, Eq. (1) takes the form of Eq. (3),
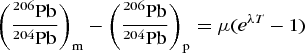 (3)
(3)where μ ≡ ()today , and the subscript m denotes a measured ratio of a representative sample. In the Pb-Pb method, the two radiometric clocks expressed in Eqs. (1) and (2) are combined to yield Eq. (4),
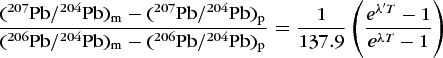 (4)
(4)where is the present ratio of , a constant reflecting the nonfractionation of the two isotopes. Primitive Pb, supposedly primordial, was found in the troilite phase of the Cañon Diablo iron meteorite, and the composition ratios of such Pb are used in Eq. (4), in which T is then the only unknown. Assuming the existence of a sample representative of the Earth as a whole, it is possible to calculate the actual age of the Earth merely on the basis of the Pb isotopic composition of such a sample. Usually what is calculated is an approximate age for the Earth, because in view of the extensive global and regional differentiation which the Earth has experienced, the assumption of a representative sample is an oversimplification.
Single-stage model
A calculation based on Eq. (4) represents a single-stage model where terrestrial Pb is assumed to have evolved (through the decay of U) in a single stage (of duration T) from an initial Pb composition identical to the nebula's primordial Pb. It is thus implicit in the model that the Earth accreted directly, or nearly so, from the solar nebula.
In his pioneering work, C. C. Patterson chose oceanic sediments as the representative sample, to which he applied Eq. (4). Repeating that calculation but using the more newly determined values for decay constants and primordial Pb yields Earth age (T) = 4.43 × 109 years and the ratio (μ) = 9.3. This terrestrial μ value is quite high when compared with estimated μ < 1 for the solar nebula and μ ∼ 0.2 inferred for the source of the Allende chondrite. If it is assumed that the Earth's average composition is chondritic (μ < 1), then it follows from the model that the high terrestrial μ is the result of chemical fractionation that took place in conjunction with the formation of the planet about 4.43 × 109 years ago.
The mechanism of such fractionation is subject to a wide range of speculations. It could be argued that the high terrestrial μ value resulted from loss of Pb by volatilization, possibly during accretion. This conjecture appears in general accord with other observations inferring depletion of the Earth in the volatiles. Alternatively, the high μ may be restricted to an outer layer, the counterpart of which is, perhaps, a deep interior sulfide region which had scavenged primordial Pb from the accreting materials. Since no terrestrial Pb reflecting low μ (say 1 to 3) has ever been sampled, it follows that convection within the Earth may be ineffective in transporting material from the deep interior. See also: Convection in the Earth; Earth's interior
If, in contrast to the above, μ ∼ 9.3 is assumed to be characteristic of the primitive material prior to its accumulation to form the planet, then the calculated age would apply to the primitive material and not necessarily to the Earth (which could have accumulated at a much later date). This possibility, however, was generally dismissed by geochronologists because it rested on the improbable premise that either the Earth accumulation and subsequent differentiation did not result in U-Pb fractionation, or chemical fractionation did occur but the resulting spectrum of μ values was averaged on the surface of the planet back to the original μ value. Not only were these implausible scenarios dismissed, but a general belief that the Earth is as old as the meteorite (4.56 × 109 years) prevailed, despite the lack of direct evidence. Subsequently, the Apollo program yielded strong evidence for the axiom of a single age for planets and meteorites: differentiated lunar rocks as old as 4.55 × 109 years were found.
Two-stage model
The axiom of 4.56 × 109 years' age applicable to planets and meteorites requires that the younger single-stage age of the Earth is an artifact resulting from the shortcomings of the model which does not take into account the length of the duration of accretion before the onsetting of U-Pb fractionation, and the multistage differentiation history of the planet.
In partial accounting for the complexities, a two-stage model may be applied and may be expressed as Eq. (5),
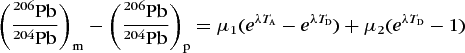 (5)
(5)where TA and TD are the times of accretion and differentiation respectively, and the subscripts 1 and 2 refer to the first and second stage. For simplicity, TA may be fixed at 4.56 × 109 years. The three unknowns (μ1, μ2, and TD) are solved for in conjunction with knowledge of the ages of uranium-free systems, for example, galenas. The two-stage age of a galena may be given by Eq. (6),
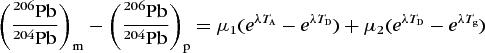 (6)
(6)where g denotes a galena which was deposited Tg years ago.
The intricate connection of the parameters is explained with help of the illustration. In this schematic presentation, 1, 2, 3, and 4 represent four meteorites falling on a straight line whose slope corresponds to 4.56 × 109 years. The farther away from primordial lead, the higher is the μ of the sample. Modern terrestrial Pb represents the average Pb composition as inferred from surface samples. The slope of the broken line corresponds to the single-stage age of the Earth as given by Eq. (4) According to the two-stage model, modern terrestrial lead did not evolve directly from primordial lead, but rather was shifted from the meteoritic line m as a result of U enrichment in a magma reservoir. The time of that differentiation event is given by the slope of the dotted line which intersects line m at X. The significance of X is that it gives the present isotopic composition of modern terrestrial lead if the differentiation event did not occur. In other words, point X would yield the correct single-stage age, given by Eq. (7).
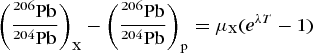 (7)
(7)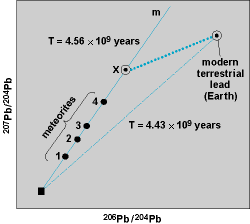
Note that μX equals μ1 of Eq. (5) [as it is the ratio of prior to the differentiation episode], and years.
The following steps are involved in the calculation: For an assumed value for TD (that is for an assumed slope for the dotted line in the illustration) the corresponding composition of undifferentiated earth (point X) is obtained from the illustration and the value of μX (that is, μ1) is calculated from Eq. (7). This is entered as μ1 into Eq. (5) from which μ2 is then calculated. Finally all three parameters, μ1, μ2 and TD are placed in Eq. (6) to yield a calculated value. The latter is compared with the known accepted deposition age, Tg, of the galena. The proper parameters are the ones which lead to .
Using these relationships in conjunction with a large number of conformable galenas and feldspars, the following parameters were calculated for Earth: TD = 3.7 × 109 years, μ1 = 7.2, and μ2 = 9.7.
Congruency of conformable galenas
The significance of the two-stage model discussed is in revealing the possibility of a global differentiation episode long after the Earth's accretion. The method of calculation, however, suffers from two main weaknesses: the age of the Earth is assumed rather than determined, and the composition of modern terrestrial lead is not a well-defined entity. In an attempt to overcome these difficulties, the so-called congruency method was put forward. The method provides a simultaneous solution to the ages of cognate galenas as well as the age of the source from which they were derived. Application of the method to conformable galenas shows that they were derived from two distinct ancient sources, A and B, whose single-stage ages are TA = 4.54 × 109 years and TB = 4.43 × 109 years. Oceanic sediments appear intimately associated with source B, which explains the young age obtained from Patterson's calculation.
Sources A and B appear to be counterparts derived from a parental source, denoted AB, through a process involving U depletion in one case (μA = 7) and its enrichment in the other (μB = 9) relative to the parental source (μAB = 8). Significantly, the indicated time of this differentiation is approximately 3.6 × 109 years ago, which appears to substantiate the first-order observation of the TD calculation. In view of such differentiation, the single-stage ages of sources A and B are gross approximations bracketing the actual age of the primary parental source AB. The age of the latter, as obtained from Eq. (4), is 4.50 × 109 years. This suggests, as an upper limit, an accretion duration of approximately 6 × 107 years prior to the onsetting of planetary differentiation resulting in the formation of the parental source.
Conclusion
There exist about half a dozen modern calculations of the Earth's age based on the Pb isotope systematics. The results fall in the narrow range of 4.43–4.57 × 109 years, yielding an average age of 4.50 × 109 years ± 0.07 × 109 years. With a dispersion of a mere ±1.5%, the age question seems to be reasonably solved, but active research in this area of geochemistry continues. The primary objective, however, is not the determination of the age with ultimate accuracy, but rather the elucidation of the evolutionary history of the planet. In particular, this type of isotopic research entails the potential of providing strict geochemical constraints which would have to be accommodated by the geophysical models. See also: Meteorite; Radioactivity; Rock age determination





