Key Concepts
A condition in space in the vicinity of an electrically charged body such that the forces due to the charge are detectable. An electric field (or electrostatic field) exists in a region if an electric charge at rest in the region experiences a force of electrical origin. Because an electric charge experiences a force if it is in the vicinity of a charged body, there is an electric field surrounding the charged body (see illustration). See also: Electric charge
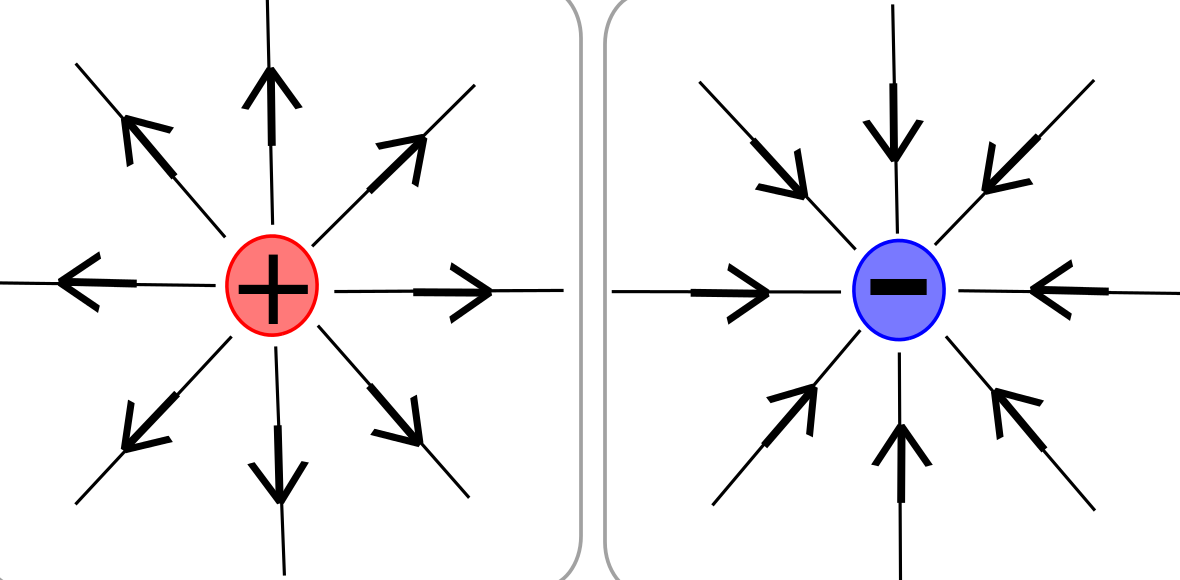
Electric fields and magnetic fields—collectively referred to as electromagnetic fields—are a manifestation of the fundamental force of nature known as electromagnetism. Electric charges with the same polarity repel each other, while opposite polarity charges attract each other. This attraction binds negatively charged electrons to positively charged atomic nuclei, forming atoms. Chemical bonds between atoms are likewise a result of electric field-mediated attraction. See also: Atom; Atomic nucleus; Chemical bonding; Electricity; Electromagnetic field; Electromagnetism; Electron; Elementary particle; Fundamental interactions
Field strength
The electric field intensity (or field strength) E at a point in an electric field has a magnitude given by the quotient obtained when the force F acting on a test charge q' (q-prime) placed at that point is divided by the magnitude of the test charge q'. Thus, field intensity E defines the force per unit charge. A test charge q' is one whose magnitude is small enough so it negligibly alters the field in which it is placed. The direction of E at the point is the direction of the force F on a positive test charge placed at the point. Therefore, E is defined as a vector point function because it has a definite magnitude and direction at every point in the field. Eq. (1) defines the electric field intensity E.
Principle of superposition
The electric field intensity (or field strength) E at a distance of r meters from a point charge of q coulombs in vacuum is given by Eq. (2),
where E is the field strength in volts per meter (V/m) and ε0 = 8.85 × 10−12 farad/m is the permittivity of free space. E is a vector, directed along the radius vector from the point charge; positive E is directed away from a positive charge or toward a negative charge. For an assembly of charges, the resultant field is, by the principle of superposition, the vector sum of the field components due to the individual charges. This summation may be performed directly, but in practical cases Gauss' theorem—named after German mathematician, physicist, and astronomer Carl Friedrich Gauss—often affords a more powerful and convenient method. See also: Coulomb's law; Gauss' theorem
Electric displacement
Electric flux density or electric displacement D in a dielectric (electrically nonconductive) material is related to E by either of the equivalent equations (3),
where P is the polarization of the medium, and ε is the permittivity of the dielectric, which is related to ε0 by the equation ε = εrε0, εr being the relative permittivity of the dielectric. In empty space, D = ε0 E. The units of D are coulombs per square meter. See also: Permittivity; Polarization of dielectrics
In addition to electrostatic fields produced by separations of electric charges, an electric field is also produced by a changing magnetic field. The relationship between the E produced and the rate of change of magnetic flux density d B/dt which produces it is given by Faraday's law of induced electromotive forces (emfs) in Eq. (4),
where d s is a vector element of path length directed along the path of integration in the general sense of E. Thus ∮E · d s is the emf induced in this closed path of integration. The area of the surface bounded by the path of integration is A, and the direction of d A, an infinitesimal vector element of this area, is the direction of the thumb of the right hand when the fingers encircle the path of integration in the general sense of E. (Note that an infinitesimal vector is a vector element which has an amplitude close to zero but is not zero.) The right side of Eq. (4) is seen to be the negative of the time rate of change of the magnetic flux (B) linking the path of integration chosen for the left side. See also: Capacitance; Faraday's law of induction; Maxwell's equations
In an electrostatic field, ∮E · d s is always zero. See also: Electromagnetic induction; Potentials





