In astronomy and astronautics, the motion of a body through space under the influence of its own inertia, the gravitational attraction of other bodies and other forces. In the early seventeenth century, Johannes Kepler discovered empirically that the orbital motions of the planets about the Sun are ellipses and Sir Isaac Newton later proved that any body subject to an inverse-square gravitational force follows a path described by a conic section, whether a circle, ellipse, parabola, or hyperbola. See also: Conic section
Two bodies interacting by the force of gravity orbit each other about their center of mass, where the less massive body follows a larger orbit. The center of mass may reside outside both bodies or within the more massive body: The center of mass of the solar system lies just outside the visible disk of the Sun, whereas the center of mass of the Earth–Moon system is approximately 1700 km (1000 mi) below the Earth's surface. See also: Center of mass
Orbital motion is typically treated by considering the relative motion of one body about another. That is, it is assumed that one body is fixed in space (usually the more massive body, sometimes called the primary) and the second orbits it. In practical applications, it is common to neglect the mass of the second body entirely if the primary is significantly larger. For example, in the case of artificial satellites, the Earth is so much more massive than a spacecraft that usually only the Earth's mass is included in calculations and the center of mass is assumed to reside at the Earth's center.
Elliptical orbits
When two bodies interact through gravity, the orbital motion occurs in a plane and most commonly in the shape of an ellipse with the primary body at a focus, as depicted in Fig. 1. For this figure, one may consider a spacecraft, S, in orbit about the Earth at the focus, F. The point P is the location on the orbit closest to the Earth and is called the perigee (specifically for the Earth, or periapsis in general); the point A is farthest from the Earth and is called the apogee (or apoapsis). A spacecraft near perigee moves more quickly than at apogee, following Kepler's second law of planetary motion, which requires a body in orbit to sweep out equal areas of the ellipse in equal times. See also: Ellipse; Kepler's laws
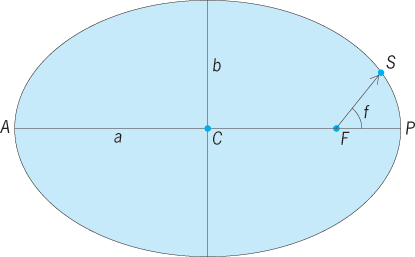
The major axis of the ellipse, AP, is called the line of apsides. The semimajor axis, a, is the half-length of the major axis and the semiminor axis, b, is the half-length of the minor axis. One complete circuit of the ellipse is an orbit and the time to complete an orbit, called the period, is proportional to the semimajor axis. In particular, the square of the period is directly proportional to the cube of the semimajor axis, per Kepler's third law.
The eccentricity of the ellipse, e, measures the deviation of the orbit from a circle and is given by the ratio e = CF/CP, where C is the center of the ellipse. For elliptical orbits, the eccentricity is always less than 1 and circular orbits have an eccentricity of 0.
For a spacecraft at S, the angle SFP (commonly denoted by f or the Greek letter ν) is called the true anomaly. The true anomaly specifies the time-dependent location of the spacecraft in its orbit. The relationship between orbital position and time is expressed by a transcendental equation, called Kepler's equation, which has no closed-form solution. See also: Kepler's equation
The orientation of the orbit plane in space is described by additional parameters. Figure 2 shows the orbit oriented in space with respect to a fundamental plane, in this case the plane of the Earth's equator, called the celestial equator. The angle between the orbit plane and the celestial equator is the orbit's inclination, i. The orbit plane intersects the celestial equator along the line of nodes, NN′. The ascending node, N, is the intersection between the orbit and the equator where the spacecraft is moving northward and the descending node, N′, is the intersection where the spacecraft is moving southward. See also: Celestial sphere
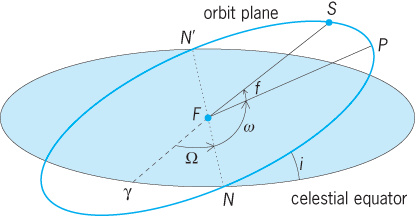
The orientation of the line of nodes in space is given by the right ascension of the ascending node, Ω, which is the angle measured from the first point in Aries, ⋎, to the ascending node. The first point in Aries denotes the intersection of the celestial equator with the ecliptic plane and is used frequently in celestial mechanics as a universal reference point. See also: Celestial mechanics; Ecliptic
The angle in the orbit plane from the ascending node, N, to the line of apsides, FP, is called the argument of perigee, ω (or, in general, the argument of periapsis). The argument of perigee determines the orientation of the orbit's line of symmetry in space.
The six parameters a, e, i, Ω, ω and f uniquely define the location of a body in orbit and are called its orbital elements. For orbital motion involving only two bodies and no other perturbing forces, the first five orbital elements are constant (that is, the shape of the orbit and its orientation in space do not change) and the sixth element, the true anomaly, increases with time and locates the body on the orbit.
Parabolic and hyperbolic orbits
Parabolic and hyperbolic orbits, which have eccentricities greater than or equal to unity, are unbounded and extend to infinity. These orbits occur infrequently in nature but are occasionally observed for comets with cosmic origins that pass through the solar system. These comets follow hyperbolic orbits with respect to the Sun. Geocentric hyperbolic orbits are necessary for artificial satellites and space probes to interplanetary destinations. Spacecraft on these orbits escape the Earth's gravitational field en route to Mars, Jupiter, or other planets and in general will not return. See also: Comet; Escape velocity; Hyperbola; Parabola
More complicated motion
Orbital motion becomes more complicated with the introduction of other external forces, including the gravity of additional bodies, the effects of the Earth's nonspherical shape, atmospheric drag and radiation pressure. These forces perturb the orbit from a conic section into a form that may not repeat and may be chaotic (that is, hard or impossible to predict over long time scales). For example, the equatorial bulge of the Earth causes both the line of nodes and the argument of perigee to drift at constant rates (called the precession of the nodes or of perigee). Atmospheric drag, which extracts energy from an orbit through friction, causes a spacecraft or passing asteroid to spiral down to (and eventually impact) the surface of a planet. See also: Chaos; Perturbation (astronomy); Radiation pressure
Modern investigations of orbital motion have discovered new classes of orbits that involve three or more bodies under the influence of gravity. The interaction of multiple gravitational fields and the judicious selection of initial conditions permit orbits in the shape of a horseshoe, triangle, square, peanut, or figure eight, among others. Many spacecraft have exploited these unique orbits for mission-related purposes, but they are also found in nature. Trojan asteroids follow peanut-shaped orbits near Jupiter, several asteroids follow horseshoe-shaped orbits with respect to the Earth and many stars follow box-shaped orbits around the center of the Milky Way. See also: Trojan asteroids





