Linear velocity

Linear velocity is velocity along a straight line, and its magnitude is commonly measured in such units as meters per second (m/s), feet per second (ft/s), and miles per hour (mi/h). A body need not move in a straight line path to possess linear velocity. The instantaneous velocity of any point of a body undergoing circular motion is a vector quantity, such as v₁ or v₂ in Fig. 2. When a body is constrained to move along a curved path (Fig. 3), it possesses at any point an instantaneous linear velocity in the direction of the tangent to the curve at that point. The average value of the linear velocity is defined as the ratio of the displacement to the elapsed time interval during which the displacement took place. The displacement of a body from an initial position s₀ to a final position s_f after time t is equal to s_f − s₀. The corresponding time interval is t_f − t₀. The magnitude of the average velocity is then given by Eq. (1),

Fig. 2 Illustration of angular displacement, angular speed, and tangential velocity.

Fig. 3 Average velocity from P₁ to P₂ is (s_f − s₀)/(t_f − t₀). The instantaneous velocity at point P is the limit of the ratio representing the average velocity as the interval approaches zero.

(1)

where Δs is displacement and Δt is the corresponding elapsed time. See also: Circle; Time

The magnitude of the instantaneous velocity v of a body is the limiting value of the foregoing ratio as the interval approaches zero. In the notation of calculus, Eq. (2), ds/dt is the instantaneous time rate of change of displacement (Fig. 3).

(2)

The velocity of a body, like its position, can only be specified relative to a particular frame of reference. Consequently, all velocities are relative. See also: Relative motion

Angular velocity

Angular velocity is the rate at which a body revolves or rotates. The representation of angular velocity ω as a vector is shown in Fig. 4. The vector is taken along the axis of spin. Its length is proportional to the angular speed and its direction is that in which a right-hand screw would move. If a body rotates simultaneously about two or more rectangular axes, the resultant angular velocity is the vector sum of the individual angular velocities. Thus, if a body rotates about an x axis with angular velocity ω_x, and simultaneously about a y axis with an angular velocity ω_y, the resultant angular velocity ω is the vector sum given by Eq. (3).

Fig. 4 Angular velocity shown as an axial vector.

(3)

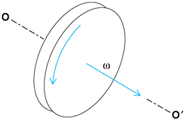
It should be emphasized that whereas angular velocities are commutative in addition, that is, they may be added in any order, angular displacements are not commutative. See also: Rotational motion

Tangential velocity

When a particle rotates in a circular path of radius R through an angular distance Δθ in a time Δt, as in Fig. 2, it traverses a linear distance Δs. The average linear speed $\bar{v}$ is given by Eq. (4),

(4)

since Δs = RΔθ. Similarly, the instantaneous speed v is given by v = ωR. The direction of this instantaneous speed is tangential to the circular path at the point in question. Any vector v drawn in this direction represents the tangential velocity.

Combined velocities

A body may have combined linear and angular motions, as is the case when the wheel of a moving automobile rolls along the ground with an angular velocity about its axle which moves with a linear velocity parallel to the pavement. In this case, a point on the rim of the tire describes a curved path called a cycloid. If a circular body rolls on the surface of a sphere, a point on the periphery of the rotating body describes a curve called an epicycloid. See also: Cycloid; Epicycloid

Vernon D. Barger