Key Concepts
The time required for one-half of a given material to undergo chemical reactions. As a term, half-life is often used in nuclear physics to quantify the average time interval required for one-half of any quantity of identical radioactive atoms to undergo radioactive decay. For example, the half-life of uranium-238 is approximately 4.5 billion years, meaning that a given quantity X will be reduced to approximately ½ X after that time interval (see illustration). The term half-life is also more broadly used in chemistry and medicine to indicate the time interval within which half of a substance will have decayed or changed in some manner. An example is the biological half-life of a drug, which refers to how many days (typically) it tends to take for biological processes to have removed half of an administered dose from the body. See also: Atom; Chemistry; Nuclear physics; Pharmacology; Radioactivity; Time; Uranium
Chemical reactions
The concept of the time required for all of a given material to react is meaningless, because the reaction goes very slowly when only a small amount of the reacting material is left and theoretically an infinite time would be required. The time for half-completion of the reaction, in contrast, is a definite and useful way of describing the rate of a reaction. See also: Chemical kinetics
The specific rate constant k provides another way of describing the rate of a chemical reaction. This is shown in a first-order reaction (1),
where c0 is the initial concentration and c is the concentration at time t. The relation between specific rate constant and period of half-life, t, in a first-order reaction is given by Eq. (2).
In a first-order reaction, the period of half-life is independent of the initial concentration, but in a second-order reaction it does depend on the initial concentration according to Eq. (3).
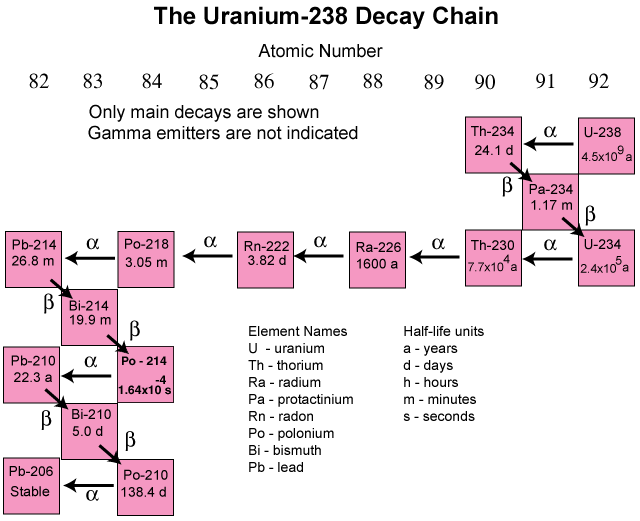
Radioactive decay
As the time it takes for one-half of the original atoms of a radioactive isotope to decay, half-life is expressed as T½ and is also called a half-period. If starting with A0 atoms at time t = 0, then the number of atoms present at a later time is given by Eq. (4),
where λ is called the decay constant. Each radioactive isotope has a unique characteristic λ. The number of atoms and the activity of the sample, given by Aλ, decrease exponentially with time. After one half-life, when A/A0 = ½, the time is given by Eq. (5), which relates the half-life to the decay constant.
After two half-lives, A/A0 = ¼; after three half-lives, A/A0 = ⅛; and so on.
Radioactive decay follows a statistical probability. The probability is exactly ½ that the actual life span of one individual radioactive atom will exceed T½. When the number of atoms is very large, then one-half that number will have decayed in T½. For a small number of initial atoms, however, the number remaining after the T½ can vary considerably around ½. See also: Probability (physics)
One common application of radioactive decay with regard to half-lives is radiometric dating, which utilizes the radioactive decay of certain long-lived, naturally occurring parent isotopes to stable daughter isotopes to measure the age of an object or sample. For example, the isotope 14C is produced in the Earth's atmosphere and has a half-life of 5700 years. Living plants take in 14C along with stable 12C. When the plant dies, the ratio decreases and can be used to tell the time when the plant died up to 10 T½ ≈ 57,000 years, but not older. Other elements and isotopes allow for dating samples back billions of years. See also: Atmosphere; Carbon; Dating methods; Isotope; Plant; Radiocarbon dating





