Key Concepts
The transport of matter from one point to another by random molecular motions. Diffusion occurs in gases, liquids, and solids.
Diffusion plays a key role in processes as diverse as permeation through membranes, evaporation of liquids, dyeing textile fibers, drying timber, doping silicon wafers to make semiconductors, and transporting of thermal neutrons in nuclear power reactors. Rates of important chemical reactions are limited by how fast diffusion can bring reactants together or deliver them to reaction sites on enzymes or catalysts. The forces between molecules and molecular sizes and shapes can be studied by making diffusion measurements. See also: Evaporation; Integrated circuits; Semiconductor; Thermal neutrons
Fluids
Molecules in fluids (gases and liquids) are constantly moving. Even in still air, for example, nitrogen and oxygen molecules ricochet off each other at bullet speeds. Molecular diffusion is easily demonstrated by dispensing a drop of ink in a beaker (Fig. 1). The boundary between the ink and water is sharp at first, but it slowly blurs as the ink diffuses upward into the clear water. Eventually, the ink spreads evenly in the beaker without any help from stirring.

Diffusion equations
Adolph Fick discovered the mathematical laws of diffusion in 1855. He found that the rate of diffusion of a substance is proportional to the gradient in its concentration (C). This is expressed in his first law, Eq. (1),
where J is the flux density, the amount of substance diffusing parallel to the z coordinate axis per unit time per unit area. The minus sign reflects the natural tendency of substances to diffuse down concentration gradients, from higher to lower concentrations. Similar equations can be written for diffusion along the x and y coordinates in three-dimensional space.
The quantity D is called the diffusion coefficient or the diffusivity. It measures the flux produced by a given concentration gradient. If the concentration is expressed in units of moles per cubic centimeter and the flux in moles per square centimeter per second, the units of D are square centimeters per second. Mass concentrations (grams per cubic centimeter) and mass fluxes (grams per square centimeter per second) are also used. Since J is the rate of diffusion per unit area, the amount of substance diffusing per second through area A is AJ.
Fick's law for the flow of matter in a concentration gradient is analogous to Fourier's law for heat conduction in a temperature gradient. See also: Conduction (heat)
Fick's second law
Diffusion changes the distribution of molecules as time passes. Each second, AJ(z) moles of substance diffuse into a slab of solution of area A and thickness Δz (Fig. 2) across its left face. Simultaneously, AJ(z + Δz) moles per second diffuse out of the slab across its right face. The net rate of diffusion into the slab is AJ(z) − AJ(z + Δz).
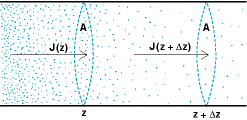
Dividing the rate of diffusion into the slab by its volume AΔz gives the rate of change of concentration, as in Eq. (2), and combining Eqs. (1) and (2)
gives Fick's second law of diffusion, Eq. (3).
As time passes, the concentration changes in proportion to the change in the concentration gradient along the diffusion path. This illustrates diffusion's fundamental role: smoothing out gradients in composition.
Diffusion coefficients can be treated as constants if the concentration gradients are not too large. The simpler version of Fick's second law, Eq. (4),
then applies. This equation is used to predict the concentrations of diffusing substances in hundreds of different applications. Slightly different equations are used for diffusion in cylindrical or spherical geometries. See also: Differential equation
Onsager's equation
Just as positive charge flows from higher to lower electric potential, molecules diffuse from regions of higher to lower chemical potential (a thermodynamic property). Gradients in the chemical potential, not concentration gradients, are the true driving forces for diffusion. A more fundamental description of diffusion states that the flux of a diffusing substance is proportional to the gradient in its chemical potential, as in Eq. (5).
The thermodynamic diffusion coefficient L is proportional to the mobility of a diffusing substance, the ease with which it moves through a fluid. Small molecules in low-viscosity fluids have the largest mobilities. Fick's diffusion coefficient D equals L∂μ/∂C. This result shows that diffusion coefficients are not purely kinetic quantities because they depend on thermodynamic properties too. See also: Chemical thermodynamics
Diffusion processes
These comprise chemical interdiffusion, self-diffusion, and intradiffusion.
Chemical interdiffusion
By far the most frequently studied diffusion process is the molecular mixing of two different chemical substances. An example is the interdiffusion of oxygen and nitrogen. At first glance, there are two diffusion coefficients to consider, one for each substance. However, there is no bulk movement of fluid during diffusion. This restriction leads to the surprising result that the interdiffusion of two substances is described by a single diffusion coefficient, D: J1 = −D∂C1/∂z and J2 = −D∂C2/∂z. The quantity D is called the interdiffusion coefficient or the mutual diffusion coefficient.
Gas molecules travel over distances of many molecular diameters before being deflected by collisions with other molecules. This gives gas mixtures the largest interdiffusion coefficients, about 0.1 cm2 s−1 at room temperature and atmospheric pressure. A gas molecule diffuses about 0.1–0.5 cm in 1 s. Diffusion in liquids is about 10,000 times slower because the molecules are packed more closely. Solid-state diffusion is even slower because the molecules are locked in position by their neighbors, except for infrequent jumps.
Self-diffusion
Diffusion occurs in pure substances too, and is called self-diffusion. It can be studied by tagging some of the diffusing molecules with isotopes. For example, the self-diffusion coefficient of water is measured by releasing trace amounts of water labeled with radioactive tritium (3H1HO) at one end of a tube filled with ordinary water (1H2O) and then monitoring the spread of radioactivity along the tube. Magnetized molecules also serve as tagged species. See also: Isotope; Nuclear magnetic resonance (NMR)
Intradiffusion
The term intradiffusion refers to the interchange of tagged and untagged species in systems of uniform chemical composition. To measure the intradiffusion coefficient of benzene in a benzene-heptane mixture containing 1 mole of benzene per liter, a 1-mole-per-liter solution of benzene in heptane is brought into contact with another 1-mole-per-liter benzene solution in which a portion of the total benzene molecules are tagged with radioactive 14C.
Gases
A number of techniques are used to measure diffusion in gases. In a two-bulb experiment, two vessels of gas are connected by a narrow tube through which diffusion occurs. Diffusion is followed by measuring the subsequent changes in the composition of gas in each vessel. Excellent results are also obtained by placing a lighter gas mixture on top of a denser gas mixture in a vertical tube and then measuring the composition along the tube after a timed interval.
Rates of diffusion in gases increase with the temperature (T) approximately as T3/2 and are inversely proportional to the pressure. The interdiffusion coefficients of gas mixtures are almost independent of the composition.
Kinetic theory shows that the self-diffusion coefficient of a pure gas is inversely proportional to both the square root of the molecular weight and the square of the molecular diameter. Interdiffusion coefficients for pairs of gases can be estimated by taking averages of the molecular weights and collision diameters. Kinetic-theory predictions are accurate to about 5% at pressures up to 10 atm (1 megapascal). Theories which take into account the forces between molecules are more accurate, especially for dense gases. See also: Kinetic theory of matter
Liquids
The most accurate diffusion measurements on liquids are made by layering a solution over a denser solution and then using optical methods to follow the changes in refractive index along the column of solution. Excellent results are also obtained with cells in which diffusion occurs between two solution compartments through a porous diaphragm. Many other reliable experimental techniques have been devised.
Room-temperature liquids usually have diffusion coefficients in the range 0.5–5 × 10−5 cm2 s−1. Diffusion in liquids, unlike diffusion in gases, is sensitive to changes in composition but relatively insensitive to changes in pressure. Diffusion of high-viscosity, syrupy liquids and macromolecules is slower. The diffusion coefficient of aqueous serum albumin, a protein of molecular weight 60,000 atomic mass units, is only 0.06 × 10−5 cm2 s−1 at 25°C (77°F).
When solute molecules diffuse through a solution, solvent molecules must be pushed out of the way. For this reason, liquid-phase interdiffusion coefficients are inversely proportional to both the viscosity of the solvent and the effective radius of the solute molecules. Accurate theories of diffusion in liquids are still under development. See also: Viscosity
Solids
Diffusion in solids is an important topic of physical metallurgy and materials science since diffusion processes are ubiquitous in solid matter at elevated temperatures. They play a key role in the kinetics of many microstructural changes that occur during the processing of metals, alloys, ceramics, semiconductors, glasses, and polymers. Typical examples of such changes include nucleation of new phases, diffusive phase transformations, precipitation and dissolution of a second phase, recrystallization, high-temperature creep, and thermal oxidation. Direct technological applications concern diffusion doping during the fabrication of microelectronic devices, solid electrolytes for battery and fuel cells, surface hardening of steels through carburization or nitridation, diffusion bonding, and sintering. See also: Creep (materials); Fuel cell; Heat treatment (metallurgy); Metal, mechanical properties of; Phase transitions; Plastic deformation of metals; Sintering; Solid-state battery; Surface hardening of steel
The atomic mechanisms of diffusion are closely connected with defects in solids. Point defects such as vacancies and interstitials are the simplest defects and often mediate diffusion in an otherwise perfect crystal. Dislocations, grain boundaries, phase boundaries, and free surfaces are other types of defects in a crystalline solid. They can act as diffusion short circuits because the mobility of atoms along such defects is usually much higher than in the lattice. See also: Crystal defects





