Fourier equation

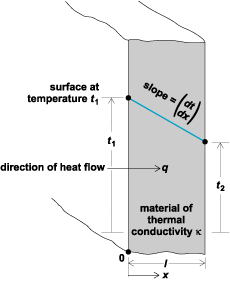
The mathematical theory, as well as the practical calculation of heat conduction, is based on a macroscopic interpretation, as contrasted to the basic microscopic mechanism just described. From a physical point of view, it is reasoned that the steady heat flow from a surface (Fig. 2) at temperature t₁ to a parallel surface at t₂ is directly proportional to (t₁ − t₂), the area A normal to the direction of flow, and the time of flow τ, and inversely proportional to the distance l between the two planes. These factors are modified by a coefficient κ accounting for the heat-conducting nature of the particular substance between the two planes. Thus, the heat flow Q (in British thermal units, for example) is given by Eq. (1).

Fig. 2 Heat flow by conduction.

(1)

In terms of the time rate of flow q = Q/τ through an infinitesimally thin layer dx, in which the temperature change is dt, this becomes Eq. (2).

(2)

The minus sign is conventionally included to make q positive when heat flows in the increasing direction of x, since dt/dx is then negative. Although this equation was first proposed by French astronomer, physicist, and mathematician Jean-Baptiste Biot (1774-1862), it is named after French mathematical physicist Joseph Fourier in honor of Fourier's extensive contributions to the theory of heat conduction.

Thermal conductivity

The coefficient κ in Eqs. (1) and (2), called the thermal conductivity, is an important property of matter. It accounts for the heat-conducting ability of a substance, and depends not only on the particular substance involved, but also on the state of that substance. The Fourier equation is essentially a definition of κ which (Fig. 2) can be interpreted as the rate of heat flow per unit of area normal to the direction of flow when a unit temperature difference exists in unit length. Thus from Eq. (2) is derived Eq. (3),

(3)

where κ is seen to have the dimensions of a heat rate per unit area and per unit of temperature gradient. In the cgs system, it can be expressed in cal/(s) · (cm²)(°C/cm), which is equivalent to cal/(s)(cm)(°C). In engineering, the units most frequently used are Btu/(h)(ft)(°F).

The thermal properties of matter are well-understood, particularly for gases, where theory involving intermolecular forces has yielded very accurate results. The process of heat conduction in liquids is believed to be similar to that of sound transmission. In dielectric solids, energy is transmitted primarily by means of waves traveling through the atomic lattice; in metals, the electrons behave like an electron gas and provide for energy transfer as well as electrical conduction. This is the basis of the Widemann-Franz law, which states that κ/σT = constant (σ is the electrical conductivity and T the absolute temperature). See also: Electron; Intermolecular forces; Kinetic theory of matter; Sound; Thermal conduction in solids

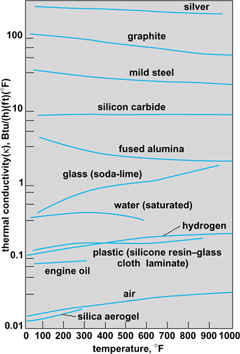
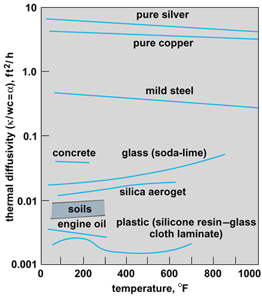
Because of the complexity of the mechanisms responsible for heat conduction, values of κ are usually determined experimentally. Results for typical gases, liquids, and solids in appropriate temperature ranges are shown in Fig. 3. The effect of pressure is significant primarily in gases.

Fig. 3 Thermal conductivities of some typical examples of gases, liquids, and solids. °C = (°F − 32)/1.8.

Differential equation of conduction

The evidence of heat flow by conduction through a substance is the variation of the temperature with location and time. If the temperature as a function of the space coordinates and time is known or can be determined, the heat flow at any location and in any direction can be specified by appropriate differentiation. A given problem is normally attacked by solving the differential equation governing the temperature distribution in a homogeneous substance and making this solution fit the prescribed initial or boundary conditions. This differential equation, essentially an expression of the first law of thermodynamics applied to the heat flow, is derived by making a heat balance on an elemental volume in a medium (Fig. 4).

Fig. 4 Diagram of the heat flow through an elemental volume in a homogeneous medium.

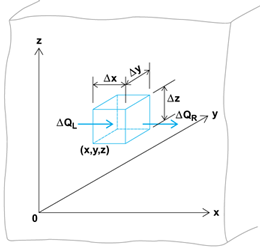
Considering first the x direction, the net heat flow into the element in time Δτ is the difference between the flowing in on the left minus that flowing out on the right; or, Eq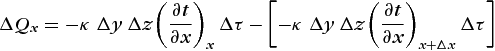. (4)

(4)

applying Eq. (1), is obtained. Accounting for the variation of κ with temperature is extremely difficult and may make the analytical solution of a problem impossible. Because of this, it is customary to use an appropriate average value which is regarded as constant. Equation (4) can then be rearranged to read as Eq. (5a ), which, as Δx → 0, becomes Eq. (5b).

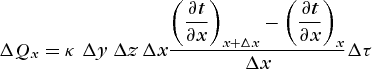(5a)

(5b)

Similar expressions apply for the y and z directions. Heat generated within the element at a uniform rate G per unit volume and time would add an amount G Δx Δy Δz Δτ.

The net heat flow into the element would be manifest as stored energy and would be equal to notation (6),

(6)

where w is the specific weight (weight per unit volume) of the medium, c its specific heat, and Δt the temperature rise in the time increment Δτ. Equating the net flow into the element to that stored and letting Δx, Δy, Δz, and Δτ → 0 leads to Eq. (7).

(7)

When no heat source is present, Eq. (7) becomes Eq. (8).

(8)

The ratio κ/wc = α is defined as the thermal diffusivity, and is the significant thermal property of a material for transient heat flow (Fig. 5). Equation (8) will be recognized as the equation governing a potential field written in terms of temperature. Similar equations are satisfied by other potential field phenomena, such as electricity, magnetism, diffusion, and ideal fluid flow. Because of this, solutions to problems in one field are applicable to analogous systems in the others. Also, an experimental solution of a problem in one field may be obtained from an analogous system in another. See also: Potentials

Fig. 5 Thermal diffusivities of some materials. °C = (°F − 32)/1.8.

Steady-state conduction

When the temperature at all locations is constant with time or, mathematically, when ∂t/∂τ = 0, steady-state conduction exists. Many important practical problems fall in this category, the most familiar being heat flow through a wall and a hollow cylinder. Referring to Fig. 2 and assuming no heat generation within the wall, Eq. (8) reduces to Eq. (9).

(9)

The terms ∂² t/∂y² and ∂² t/∂z² are eliminated, since t is considered to vary only with x. The desired temperature distribution is obtained by integrating Eq. (9) twice and evaluating the two constants from the known temperatures at x = 0 and x = 1. This leads to Eq. (10). Application of Eq. (2) to obtain q yields Eq. (11).

(10)

(11)

In the case of steady radial heat flow through a cylindrical wall (Fig. 6), Eq. (2) is applicable to any imaginary thin annular ring in the wall; thus Eq. (12)

Fig. 6 Steady-state conduction. Diagrams show the temperature distribution for steady radial heat flow through a circular cylinder wall.

(12)

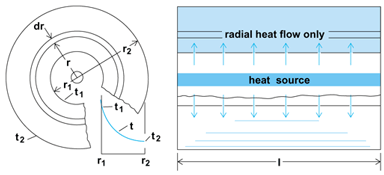
is obtained. Integration from t = t₁ to t, and r₁ to r leads to Eq. (13),

(13)

which indicates that the effect of the increasing area for heat flow is to produce a logarithmic variation in the temperature, as shown by the curve in the left part of Fig. 6.

Heat flow through a cylindrical wall is usually expressed in the same form as Eq. (11), written as Eq. (14). Solving Eq. (13) for q and substituting in Eq. (14)

(14)

shows that the appropriate value for A_m must be as given in Eq. (15),

(15)

which is called the logarithmic-mean area.

If the rate of heat flow and the inner and outer temperatures of a plane wall or hollow cylinder are measured during steady heat conduction, values of κ can be determined from Eq. (11) or Eq. (14). Because of the simplicity of the equations and the physical systems, most devices for measuring thermal conductivity are based on these types of heat flow.

Interface resistance

Now consider steady-state heat flow through a wall composed of two or more layers of material, each with different uniform thermal properties. If the surfaces of the various layers are very smooth and in very good contact with each other, the temperature distribution will be continuous. At any interface (Fig. 7a), since q is constant, Eq. (16)

Fig. 7 Temperature distribution through composite wall. (a) With perfect interface contact. (b) For typical actual surfaces.

(16)

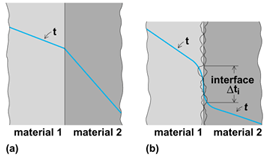
holds. This shows that there is a discontinuity in the temperature gradient due to the change in κ.

Actual surfaces, even polished ones, are not smooth but have small projections and depressions. Consequently, when two surfaces are brought together, contact occurs primarily at projecting spots, as illustrated in Fig. 7b. The resulting contact area is only a small fraction of the nominal contact area. Plastic deformation at the points of contact of one or both materials usually occurs with the application of force to hold them together. Heat flows through both the small contact areas and the substance (usually a gas or liquid) filling the voids between the contacting protuberances.

The impairment to the heat-flow path caused by this imperfect contact is referred to as contact resistance. This is determined by extrapolating the measured temperature distribution in each material to the apparent interface location (Fig. 7b). The quotient of the resulting temperature difference Δt_i thus determined and the heat flux defines an interface resistance R_i = $\text{Δ}t{}_i\text{/}q$. R_i depends on the roughnesses of the surfaces, the gas or liquid filling the voids, and the contact pressure. In general, the effect of contact resistance is significant only at low (for example, below 100 lb/in.² or 700 kPa) interface pressures.

Periodic and transient conduction

These are the two kinds of non-steady-state heat flow. Periodic means a quasi-steady-state condition in which the temperature and heat flow at any location in a body vary continuously with time, but pass through the same series of values in a definite period of time, τ₀. A transient state results when the heat flow at any location is momentarily or permanently changed. The duration of the transient period is the time required for the system to return to its original or a new steady-state condition. A transient change may be superimposed on a periodic variation.

Restricting consideration to examples in which no heat generation is present, the fundamental differential equation to be satisfied for either periodic or transient heat flow is Eq. (8). An interesting application is the temperature variation in the Earth due to the diurnal temperature variation, or to a sudden change in surface temperature. Equation (8) reduces in this case to Eq. (17).

(17)

Assume the existence of a mean temperature of the Earth which is invariable with depth and that its surface temperature has been varying in a steady periodic manner long enough so that the original transient state due to starting the cyclic surface temperature has reached a steady periodic condition. If the surface temperature variation is given by Eq. (18), the appropriate solution to Eq. (17) is given by Eq. (19).

(18)

(19)

This result shows that the temperature distribution looks like a wave traveling into the medium with the amplitude decreasing as the factor e^{-$\sqrt{\raisebox{1ex}{$\text{π}$}\!\left/ \!\raisebox{-1ex}{$\text{2γ}$}\right.}$ x}. Computation of the heat flow by determining (∂t/∂t)_{x = 0} from Eq. (19) indicates that heat flows in during one-half of the period τ₀ and out during the other half.

Calculations of the temperature distribution in the Earth over an 8-h interval, using Eq. (19), are shown in Fig. 8. In this case the surface temperature varied from 29 to 41°F (−1.6 to 5.0°C) over a 24-h period; the diffusivity of the soil was 0.0065 ft²/h (6.0 × 10⁻⁴ m²/h).

Fig. 8 Temperature distribution in the ground for a daily periodic surface variation. °C = (°F − 32)/1.8.

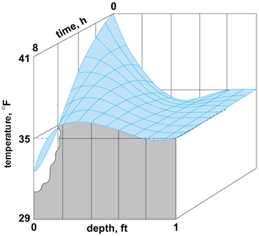
An illustration of a transient state is the temperature variation resulting from a sudden change of magnitude t₀ in the temperature at the surface of the Earth. When the initial temperature throughout is uniform and taken as the datum, the applicable solution to Eq. (17) is given by Eq. 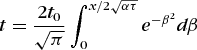(20).

(20)

If a body of soil in the Earth's surface is at a uniform temperature of 40°F (4.4°C) and the surface temperature suddenly drops to 20°F (−6.7°C), Eq. (20) can be applied to determine the depth at which the temperature will have dropped to freezing (32°F or 0°C) in 12 h. Taking α = 0.0065 ft²/h, t = 32°F after 12 h is found to occur at x = 0.27 ft = 3.2 in. = 8.2 cm.

Variable thermal conductivity

Most materials are sufficiently homogeneous so that their thermal properties are independent of position. Assuming that they vary only with temperature, the heat balance on the element of Fig. 4 leads to Eq. (21). Upon carrying out the indicated differentiation, Eq. (21) becomes Eq. (22). By introducing a new variable θ called the conductivity potential, notation (23), Eq. (22) can be simplified to Eq. (24).

(21)

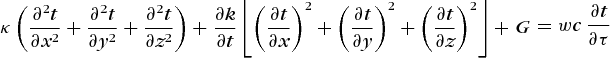(22)

(23)

(24)

Equation (24) has exactly the same form as Eq. (7). In many cases the variation of α with temperature (Fig. 5) is less than that of κ so that a mean constant value may be selected. This is true, for example, of metals at temperatures near absolute zero. In such cases, if G is not a function of t (or no heat source is present), solutions for constant α will apply with θ replacing t, provided that the boundary conditions are specified in terms of t or κ(∂t/∂n) where n represents the variables x, y, z.

The utility of the conductivity potential is demonstrated in the calculation of the heat leak to a liquid-nitrogen tank through a support rod. Consider steady-state conditions with the exposed end of the rod at 300 K and the other at 77.3 K. Also assume there are no losses from the side of the rod which is 1 cm² in cross section, is 15 cm long, and is made of stainless steel (for which κ decreases from 0.15 at 300 K to 0.08 W/cm K at 77.3 K). At any location q is given by Eq. (25). Since q is constant, Eq. (26) holds. Then Eq. (27) follows.

(25)

(26)

(27)

Warren H. Giedt