Key Concepts
The most basic, irreducible forces that act between elementary particles, of which all matter is assumed to be composed. The fundamental interactions describe how matter behaves on the smallest subatomic scales and the largest cosmological scales (Fig. 1). See also: Atom; Elementary particle; Matter (physics); Universe
Properties of interactions
At present, four fundamental interactions are distinguished. The properties of each are summarized in the table.
| Interaction | Range | Exchanged quanta |
|---|---|---|
| Gravitational | Long-range | Gravitons (g) [hypothetical] |
| Electromagnetic | Long-range | Photons (γ) |
| Weak nuclear | Short-range ≈ 10−18 m | W+, Z0, W− |
| Strong nuclear | Short-range ≈ 10−15 m | Gluons (G) |
Gravitational interaction
The gravitational interaction, more familiarly known as gravity, manifests itself as a long-range force of attraction between all elementary particles. The best description of gravity is general relativity, proposed by German-born U.S. theoretical physicist Albert Einstein in 1915. Attempts to quantum mechanically describe gravity, which include the theory of quantum gravity, propose fundamental particles known as gravitons as carriers of gravitational force. The existence of the graviton, however, has not yet been experimentally demonstrated. See also: Graviton; Gravity; Quantum gravitation; Relativity
In classical mechanics, the force law between two particles of masses m1 and m2 separated by a distance r is well approximated by the Newtonian expression ), where GN is the Newtonian constant, equal to 6.6742 ± 0.0010 × 10−11 m3 · kg−1 · s−2. The dimensionless quantity is usually taken as the constant characterizing the gravitational interaction, where me, and mp are the electron and proton masses, 2πℏ is Planck's constant, and c is the velocity of light. See also: Light; Mass; Newton's laws of motion; Planck's constant
Electromagnetic interaction
This interaction is responsible for the long-range force of repulsion of like, and attraction of unlike, electric charges. The dimensionless quantity characterizing the strength of electromagnetic interaction is the fine-structure constant, given by Eq. (1) in SI units, where e is the electron charge and ∊0 is the permittivity of empty space.
At comparable distances, the ratio of gravitational to electromagnetic interactions (as determined by the strength of respective forces between an electron and a proton) is given by the quantity , which is approximately 4 × 10−37. See also: Coulomb's law; Electrostatics
In modern quantum field theory, the electromagnetic interaction and the forces of attraction or repulsion between charged particles are pictured as arising secondarily as a consequence of the primary process of emission of one or more photons (particles or quanta of light) emitted by an accelerating electric charge (in accordance with Maxwell's equations) and the subsequent reabsorption of these quanta by a second charged particle. The spacetime diagram (introduced by U.S. physicist Richard Feynman) for one photon exchange is shown in Fig. 2. A similar picture may also be valid for the gravitational interaction (in accordance with the quantum version of Einstein's gravitational equations), but with exchanges of zero-rest-mass gravitons (g) rather than zero-rest-mass photons. See also: Feynman diagram; Photon; Rest mass; Spacetime

In accordance with this picture, the electromagnetic interaction (to one photon exchange approximation) is usually represented by reaction (2), where γ is the photon, emitted by the electron and reabsorbed by the proton.
For this interaction, and also for the gravitational interaction represented by reaction (3), the nature of the participating particles (electron e and proton P)
is the same, before and after the interaction, and the exchanged quanta (γ or g) are electrically neutral. See also: Electron; Electromagnetic radiation; Electromagnetism; Maxwell's equations; Photon; Quantum electrodynamics; Quantum field theory; Quantum mechanics
Weak nuclear interactions
The third fundamental interaction is the weak nuclear interaction, which is responsible for the decay of a neutron into a proton, an electron, and an antineutrino. Its characteristic strength for low-energy phenomena is measured by the Fermi constant GF, which is equal to 1.0268 × 10−5 mp−2ℏ3/c. Unlike electromagnetism and gravitation, weak interactions are short-range, with a force law of the type e−MWcr/ℏ, the range of the force () being of the order of 10−18 m. See also: Weak nuclear interactions
Until 1973, the only known weak interactions were those which changed the nature of the interacting particles (unlike electromagnetism and gravity). For example, consider reactions (4), where P is the proton,
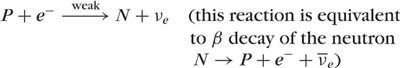 (4a)
(4a) (4b)
(4b)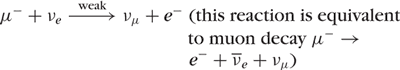 (4c)
(4c)N is the neutron, μ− is the negatively charged muon, νe and νμ are the electronic and muonic neutrinos. In reaction (4a), the weak interaction transforms a proton into a neutron and at the same time an electron into a neutrino. See also: Neutrino; Neutron
An important question was finally answered in 1983: Is the weak interaction similar to electromagnetism in being mediated primarily by intermediate objects, the W+ and W− particles? If this is the case, then reactions (4a ) and (4c), for example, would in detail be represented as reactions (5a) and (5b).
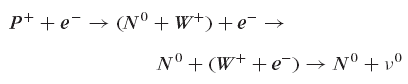 (5a)
(5a)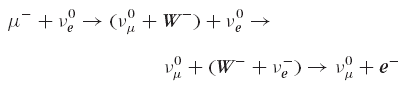 (5b)
(5b)[The superscript on each particle gives its electrical charge (+, 0, −) in units of the proton's charge.] The experimental answer (discovered at the CERN laboratory at Geneva) is that W+ and W− do exist, with a mass mW of 80.4 GeV/c2. Each carries a spin of magnitude ℏ just as does the photon (γ). The mass of these particles gives the range [ = 10−18 m] of the weak interaction, and is also related to its strength GF, as discussed below. See also: Intermediate vector boson; Spin (quantum mechanics)
Another crucial discovery in weak interaction physics was the neutral current phenomenon in 1973, that is, the discovery of new types of weak interactions where (as in the case of electromagnetism or gravity) the nature of the interacting particles is not changed during the interaction, as in reactions (6).
See also: Neutral currents
The 1983 experiments at CERN also gave evidence for the existence of an intermediate particle Z0 which is believed to mediate such reactions. Thus reaction (6a), expressed in detail, is reaction (7).
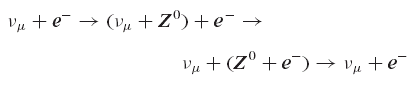 (7)
(7)The mass mZ of the Z0 has been found to be 91.2 GeV/c2. The magnitudes of the W+, W−, and Z0 masses had been predicted by the unified theory of electromagnetic and weak interactions (the electroweak interaction, discussed below), 16 years before the experiments that discovered them.
In contrast to gravitation, electromagnetism, and strong nuclear interactions, weak interactions violate left-right and particle-antiparticle symmetries. See also: CP symmetry and its violation; Parity (quantum mechanics); Symmetry laws (physics)
Strong nuclear interaction
The fourth fundamental interaction is the strong nuclear interaction between protons and neutrons, which resembles the weak nuclear interaction in being short-range, although the range is of the order of 10−15 m rather than 10−18 m. Within this range of distances the strong force overshadows all other forces between protons and neutrons, with a characteristic strength parameter of the order of unity (compared with the electromagnetic strength parameter α ≈ ).
Protons and neutrons are themselves made up of yet more fundamental entities, the up (u) and down (d ) quarks (P = uud, N = udd ). Each quark is assumed to be endowed with one of three color quantum numbers [conventionally labeled red (r), yellow (y), and blue (b)]. The strong nuclear force can be pictured as ultimately arising through an exchange of zero rest-mass color-carrying quanta of spin ℏ called gluons (G) [analogous to photons in electromagnetism], which are exchanged between quarks (contained inside protons and neutrons), as in reaction (8).
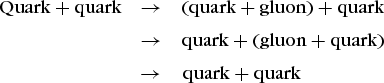 (8)
(8)Since neutrinos, electrons, and muons (the so-called leptons) do not contain quarks, their interactions among themselves or with protons and neutrinos do not exhibit the strong nuclear force. There is indirect experimental evidence for the existence of the gluons and of their spin being ℏ. See also: Color (quantum mechanics); Gluons; Lepton; Quantum chromodynamics; Quark; Strong nuclear interactions
Gauge interactions
Three of the four fundamental interactions (electromagnetic, weak nuclear, and strong nuclear) are mediated by intermediate quanta (photons γ; W+, Z0, and W−; and gluons G, respectively), each carrying spin of magnitude ℏ. This is characteristic of the gauge interactions, whose general theory was given by German mathematician and mathematical physicist Hermann Weyl, Chinese physicist Yang Chen Ning, U.S. physicist Robert Mills, and British mathematical physicist Ronald Shaw. This class of interactions is further characterized by the fact that the force between any two particles (produced by the mediation of an intermediate gauge particle) is universal in the sense that its strength is (essentially) proportional to the product of the intrinsic charges (electric, or weak-nuclear, or strong-color) carried by the two interacting particles concerned.
The fourth interaction (the gravitational) can also be considered as a gauge interaction, with the intrinsic charge in this case being the mass; the gravitational force between any two particles is proportional to the product of their masses. The only difference between gravitation and the other three interactions is that the gravitational gauge quantum (the graviton) carries spin 2ℏ rather than ℏ. As discussed below, it is an open question whether all fundamental interactions are gauge interactions. See also: Gauge theory
Unification of interactions
Ever since the discovery and clear classification of these four interactions, particle physicists have attempted to unify these interactions as aspects of one basic interaction between all matter. The work of English physicist and chemist Michael Faraday and Scottish physicist James Clerk Maxwell in the nineteenth century, which united the distinct forces of electricity and magnetism as aspects of a single interaction (the gauge interaction of electromagnetism), has served as a model for such unification ideas.
Gravitation and electromagnetism
The first attempt in this direction was made by Einstein who, having succeeded in understanding gravitation as a manifestation of the curvature of spacetime, tried to comprehend electromagnetism as another geometrical manifestation of the properties of spacetime, thus achieving a unification between these forces. In this attempt, to which he devoted all his later years, he is considered to have failed.
Electroweak interaction
A unification of weak and electromagnetic interactions, employing the gauge ideas discussed above, was suggested by U.S. particle physicist Sheldon Lee Glashow and by Pakistani theoretical physicist Abdus Salam (the author of this article) and British-Australian physicist John Clive Ward in 1959. This followed a parallel between these two interactions, pointed out by U.S. physicist Julian Schwinger in 1957. Assuming that (the then known) weak interactions (4) were mediated by exchanges of (the then hypothetical) W+ and W− particles, it could be shown from the empirical properties of weak interaction phenomena, that if the W's existed, they must carry an intrinsic spin of magnitude ℏ, just as does the photon, the gauge quantum of electromagnetism. If a bold unifying assumption was made that this magnitude of spins ℏ for W+, W−, and the photon γ connotes a gauge character for a unified electroweak interaction, and that the intrinsic coupling strength of weak interactions is universally the same as that for electromagnetism (that is, α = ), then it could be shown that the masses of the W+ and W− particles must be in excess of the quantity given in Eq. (9).
Following this initial attempt, Glashow (and independently Salam and Ward) noted that such a unification hypothesis is incomplete, inasmuch as electromagnetism is a left-right symmetry-preserving interaction, in contrast to the weak interaction, which violates this symmetry. A gauge unification of such disparate interactions could be effected only if, additionally, new weak interactions represented by reactions (5) are also postulated to exist. Equivalently, there must exist a new electrically neutral intermediate weak-quantum Z0 besides the (hypothetical) W+ and W−.
Spontaneous breaking and renormalization
There were two major problems with this unified electroweak gauge theory considered as a fundamental theory. Yang and Mills had shown that masslessness of gauge quanta is the hallmark of unbroken gauge theories. The origin of the masses of the weak interaction quanta W+, W−, and Z0 (or equivalently the short-range of weak interactions), as contrasted with the masslessness of the photon (or equivalently the long-range character of electromagnetism), therefore required explanation. The second problem concerned the possibility of reliably calculating higher-order quantum effects with the new unified electroweak theory, on the lines of similar calculations for the “renormalized” theory of electromagnetism elaborated by Japanese physicist Shin'ichirō Tomonaga, Schwinger, Feynman, and English-born U.S. physicist Freeman Dyson around 1949. The first problem was solved by U.S. theoretical physicist Steven Weinberg and Salam and the second by Dutch theoretical physicist Gerard t'Hooft and by Korean-born U.S. theoretical physicist Benjamin Lee and French theoretical physicist Jean Zinn-Justin. See also: Renormalization
Weinberg and Salam considered the possibility of the electroweak interaction being a “spontaneously broken” gauge theory. By introducing an additional self-interacting Higgs-Englert-Brout-Kibble particle into the theory, they were able to show that the W+, W−, and Z0 would acquire well-defined masses through the so-called Higgs mechanism, these masses being given by Eqs. (10),
where 37.4 GeV/c2 is the combination of constants given by Eq. (9). Here θw is a weak mixing parameter for electromagnetism and weak interactions. The constant sin2 θw can be determined from experiments which give the ratios of cross sections of Z0-mediated reactions (6) to the W+ and W−-mediated reactions (4). The best available value, calculated from all low-energy experiments, is given by Eq. (11).
See also: Symmetry breaking
The predicted theoretical mass values of the W and Z particles deduced by substituting Eq. (11) into Eqs. (10) are in good accord with the experimental values found by the CERN 1983 experiments. The existence of the W and Z particles and this accord with regard to mass values give support to the basic correctness of the electroweak unification ideas, as well as to the gauge character of the electroweak interaction.
Higgs particle
The Weinberg-Salam electroweak theory contained an additional neutral particle (the Higgs boson) but did not predict its mass. A search for this particle commenced at multiple particle accelerators, culminating in the 2012 discovery of the Higgs at the Large Hadron Collider at CERN. See also: Electroweak interaction; Higgs boson; Higgs boson detection at the LHC; Large Hadron Collider (LHC); Particle accelerator; Standard model
Electronuclear interaction
The gauge unification of weak and electromagnetic interactions, which started with the observation that the relevant mediating quanta (W+, W−, Z0, and γ) possess intrinsic spin ℏ, can be carried further to include strong nuclear interactions as well, if these strong interactions are also mediated through quanta (gluons) carrying spin ℏ. The resulting theory, which appears to explain all known low-energy phenomena, is called the standard model. (It is a model based on three similarly constituted generations of quarks and leptons plus the mediating quanta W+, W−, Z0, photons, and gluons plus the Higgs particle.) See also: Standard model
A complete gauge unification of all three forces (electromagnetic, weak-nuclear, and strong-nuclear) into a single electronuclear interaction seems plausible. Such a (so-called grand) unification necessarily means that the distinction between quarks on the one hand and neutrinos, electrons, and muons (leptons) on the other, must disappear at sufficiently high energies, with all interactions (weak, electromagnetic, and strong) clearly manifesting themselves then as facets of one universal gauge force with a primitive universal strength equal to α/sin2 θw. The fact that at low energies presently available, these interactions exhibit vastly different effective strengths is ascribed to differing renormalizations due to successive spontaneous symmetry breakings. A startling consequence of the eventual universality and the disappearance of distinction between quarks and leptons is the possibility, first discussed by Indian-born U.S. theoretical physicist Jogesh Pati and Salam within their electronuclear model, of protons transforming into leptons and pions. Contrary to the older view, protons would therefore decay into leptons and pions and not live forever. The discovery of proton instability (with decays into leptons or antileptons) would be an epic discovery and a direct confirmation of the electronuclear (grand) unification. To date, experiments have not witnessed proton decay, having established the proton lifetime as longer than 1.6 x 1034 years. See also: Grand unification theories; Proton
Consequences of symmetry breaking
Spontaneous symmetry breaking of gauge interactions has the characteristic that symmetry breaking is a phase phenomenon and disappears in a high-temperature environment. This implies that, at temperatures T in excess of 1015K (T greater than , where k is the Boltzmann constant), that is, up until 10−12 s after the outset of the big bang, there was no spontaneous breaking of the symmetry of electroweak interactions, and the W and the Z particles were massless, like the photons and the gluons. The onset of such phase transitions plays a crucial role in modern cosmological theories of the early universe, resolving some old dilemmas. For example, proton decay, and left-right and particle-antiparticle symmetry violations, provide a natural explanation for the fact that the present universe contains a preponderance of protons and neutrons rather than of their antiparticles. However, the existence of such phase transitions also poses some new dilemmas, such as the prediction of the existence of heavy magnetic monopoles (in the early universe), with abundances surviving into the present epoch, for which there is no experimental evidence. To remedy this, it is necessary to postulate an inflationary epoch having occurred in the universe's history somewhere about 10−33 s after the onset of the big bang. See also: Antimatter; Big bang theory; Cosmology; Inflationary universe cosmology; Magnetic monopoles; Phase transitions; Symmetry breaking; Symmetry laws (physics); Universe
Prospects for including gravity
Research in unification theories of fundamental interactions is now concerned with uniting the gauge theories of gravity and of the electronuclear interactions. One promising approach is the extension of spacetime to more than four dimensions, following ideas developed by German mathematician and physicist Theodor Kaluza and Swedish theoretical physicist Oskar Klein in the 1920s. Remarkably, the formal expression for Einstein's gravitational interaction in a spacetime of dimensions higher than four, is equivalent to the standard Einstein theory of spin-2ℏ gravitons in four dimensions plus a Yang-Mills theory of spin-ℏ particles (that is, a theory describing the electronuclear type of gauge interactions) when the extra dimensions are contracted down to less than 10−35 m. No realistic model of such a compactified unified theory has emerged, though Einstein-like supersymmetric theories in 10-space and 1-time (a total of 11 dimensions) are the favored candidates. (Supersymmetry is the principle which treats gauge and Higgs particles on a par with quarks and leptons.) See also: Supergravity; Supersymmetry
The most promising approach appears to be that of superstring theories. Such theories appear to describe the only possible theory of gravity which is finite and suffers from no ultraviolet infinities. A closed string is a (one-dimensional) loop which may exist in a d-dimensional spacetime (where d must equal 10 to completely eliminate all ultraviolet infinities). The quantum oscillations of the string correspond to particles of higher spins and higher masses, which may be strung on a linear trajectory in a spin-versus-mass2 (Regge) plot. Among these are the zero-mass gravitons and the gauge mesons. The theory has a unique built-in gauge symmetry.
So far, it has not been possible to go down from d = 10 dimensions to d = 4 conventional spacetime dimensions and to produce the emergence of the standard model, although there is hope that this may be accomplished. If these string ideas are successful, they may help lead to one single theory which unites all known low-energy phenomena. See also: Superstring theory





