Key Concepts
The basic principles of classical dynamics. Newton's laws of motion are three fundamental principles that form the basis of classical, or Newtonian, mechanics (Fig. 1). These laws have proved valid for all mechanical problems not involving speeds comparable with the speed of light and not involving atomic and subatomic particles. See also: Classical mechanics; Light; Motion; Relative motion; Relativity

Newton's laws of motion are as follows:
- A body not acted on by a force moves with constant velocity, that is, with constant speed in a straight line. See also: Force; Speed; Velocity
- The acceleration (rate of change of velocity with time) of a body is proportional to the force acting on it, and inversely proportional to its mass. For precision, if the body is large and irregular or soft, the words “of a body” must be replaced by "of the center of mass of a body." See also: Acceleration; Center of mass; Mass
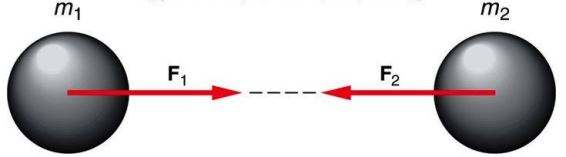
- Two bodies exert forces on one another that are equal in magnitude, but opposite in direction (Fig. 2).
The first law is merely a special case of the second law when the force is zero.
The second law (Fig. 1) is usually stated as "force equals mass multiplied by acceleration," F = m a, or as "force equals the rate of change of momentum." Although this statement is often regarded as merely defining the force F on a body given its mass m and acceleration a, the fact drawn from experience by Isaac Newton is that a nonzero force always has a discernible reason, namely, interaction with one or more other bodies, for example, by gravitational attraction. Given the dependence of the forces on the locations of the bodies (for example, the inverse-square law of gravity), then the collection of F = m a equations for the bodies is an equation of motion (technically, a set of coupled second-order differential equations), from which the motion of the bodies can be calculated, given their locations and velocities at one time. See also: Differential equation; Gravitation; Linear momentum; Momentum
The third law (Fig. 2), often referred to as "action equals reaction," has the important consequence that all the many internal forces exerted by parts of a body on one another are irrelevant to the motion of the body because they cancel out. The effects of quantum mechanics and special relativity on the motions of the parts, no matter how light or rapidly moving they are, are also irrelevant as long as the body itself is sufficiently heavy and slowly moving. However, the third law is not always true in Newton's sense; for example, the magnetic forces between moving charged bodies do not obey the third law because the electromagnetic field can carry momentum. See also: Conservation of momentum; Electromagnetic field; Magnetism; Rigid body
There also is a rotational analog of the second law (the force on a body equals the rate of change of its momentum), namely, that the torque on a body equals the rate of change of its angular momentum. However, there is no analog to the first law, except in the very special case of rotation of a rigid body around a fixed axis. See also: Angular momentum; Rigid-body dynamics; Torque
Newton was well aware that there is no way to measure “absolute” motion of a body, that is, without comparison to the motion of some other material thing. One can measure the motion relative to oneself (“the observer”), but two observers who are accelerating or rotating relative to one another will see the acceleration of a body to be different, and hence they will not both see Newton's laws obeyed. An observer who sees Newton's laws obeyed is called “inertial.” It is important to note that the velocities (although not the accelerations) of such observers may be different; this is usually called Galilean relativity. Einstein's special relativity is the modification that allows electromagnetic phenomena to be included in this relativity of motion. See also: Frame of reference; Galilean transformations





