Key Concepts
Any interaction between objects that causes motion. Fundamentally, objects interact through the four known physical forces: gravity, electromagnetism, and the strong and weak nuclear interactions. In practice, however, many of the forces worked with when solving everyday problems are macroscopic manifestations of these four fundamental interactions that take place at the level of subatomic particles. For example, the macroscopic contact force between two objects—typically called the normal force—is fundamentally an electrostatic interaction between the electron clouds of molecules at the interface of the two objects. At the introductory physics level, though, it is typically not useful to describe the interaction in fundamental terms, and normal force considerations are sufficient (Fig. 1). In this way, Newton's laws—first postulated by English physicist and mathematician Isaac Newton—provide an essential framework for understanding how forces determine the motion of objects. While widely applicable in most situations, Newton's laws fail to describe motion in certain, less-frequently-encountered circumstances, for instance involving objects moving close to the speed of light or in non-inertial reference frames. These circumstances require application of the special and general theories of relativity, respectively. See also: Electromagnetism; Fundamental interactions; Gravity; Newton's laws of motion; Relativity; Strong nuclear interaction; Weak nuclear interaction
Newton's laws
Newton's first law states that an object in uniform motion (not accelerating), will remain in uniform motion unless acted upon by a force. This means that an object with constant velocity (including an object at rest, with constant velocity of zero), would otherwise remain in that state of motion indefinitely. An object can remain in motion with no net force acting on it, but force is necessary to cause a change in its motion, resulting in increased or decreased speed, and/or change in direction. Without force there can be no acceleration. See also: Acceleration; Motion
Newton's second law provides a concrete mathematical relation between the force on an object and its resultant acceleration:
where F is the total force exerted on an object, m is the mass of the object, and a, the object's acceleration. (Note: This article follows the convention of using bold, non-italicized variables for vectors and using non-bold, italicized variables for scalars.) Thus, the larger the mass of an object, the less it will accelerate under the influence of a given force. (Note: Newton originally state this law in terms of the time rate of change of the object's momentum p, as F = , which is equivalent to Equation (1) for an object of constant mass.) Equation (1) is a vector equation, meaning the direction of the force and acceleration matter: F produces acceleration a, in the same direction. If an object is in uniform motion with velocity v in the same direction as F, v will increase. If F is perpendicular to v, the direction, but not the magnitude of v will change as a result of F. In the special case where F remains perpendicular as v changes the resultant acceleration is called centripetal acceleration and the result is uniform circular motion. It is also important to recognize that F in Equation (1) is the total, or net, force acting on the object. It is often the case that a force acting on an object is opposed by another force such that the net force (and therefore the acceleration) is zero. See also: Mass; Momentum
Newton's third law states that for a pair of objects exerting force on each other, the force exerted on one is equal in magnitude and opposite in direction to the force exerted on the other. As shown in Fig. 2, for two objects 1 and 2 attracted by the force of gravity (or any other attractive force), the force on 1 due to 2, F1,2 , is equal in magnitude and opposite in direction to the force on 2 due to 1, F2,1 .
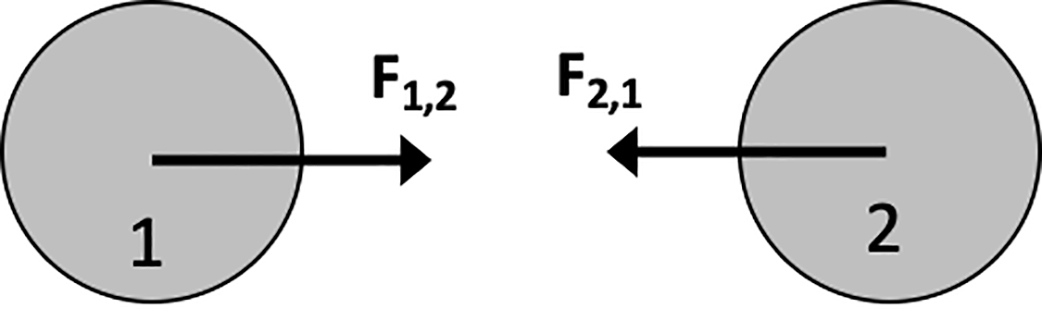
These force pairs are always equal and opposite, regardless of the mass of the two objects. For example, the Earth exerts a gravitational force on the Sun, which is equal in magnitude and opposite in direction to the gravitational force exerted on the Earth by the Sun. This may appear counterintuitive, but if combined with Newton's second law, it becomes clear that the acceleration of the Sun due to the force exerted by the Earth is tiny compared to the acceleration of the Earth, because the mass of the Earth is very small compared to the mass of the Sun. Hence, the Earth orbits the Sun, not the other way around. See also: Earth; Orbital motion; Sun
Solving problems with forces
Free body diagrams
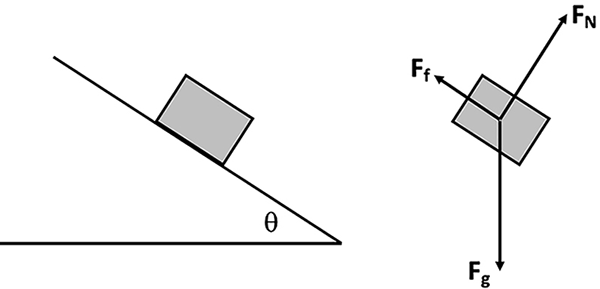
When solving problems with Newton's second law, a free body diagram is often used to identify all the forces acting on an object and the direction of each force. For example, consider a block at rest on an inclined plane that makes an angle Θ with the horizontal as shown in Fig. 3. The block itself is separated from its surroundings, and shown as a free body, on the right side of the figure. There are three forces acting on the block: the force of gravity (Fg ), the normal force exerted on the block by the plane (FN ), and a frictional force (Ff ) exerted by the surface of the plane on the bottom surface of the block. The direction and the approximate magnitudes of each force are indicated by the arrows in the free body diagram. See also: Friction; Vector methods
Equations of motion
Once each force acting on an object has been identified, we can set up an equation of motion for that object by inserting the sum of all forces into Equation (1). For problems with forces acting in more than one dimension (such as the inclined plane in Fig. 3), it is necessary to break forces down into their components along each coordinate axis. The sum of the forces acting along that coordinate axis are written on the left side of the equation, and the corresponding acceleration component (e.g. ax and ay ) are substituted on the right side of the equation. This typically requires choice of an appropriate coordinate system and use of trigonometric functions to determine the projection of each force onto those coordinate axes. See also: Trigonometry
Conservative and non-conservative forces
It is important to distinguish between conservative forces, such as the force of gravity, and non-conservative forces, such as frictional drag forces, both of which are frequently encountered in elementary physics problems. A conservative force has the mathematical property that the vector function describing the force at any point in space, F(x, y, z), can be written as the gradient of a scalar function, called a potential, U(x, y, z):
An important consequence of this equation is that the mechanical work done by a conservative force is independent of the path taken. For example, if an object is dropped from a certain height off the ground and then lifted back up to the height at which it started, the total work done by the force of gravity (which is conservative) is zero. By returning the object back to its original height, the force of gravity once again has potential to do mechanical work on the object. This is an important property, which is closely related to how potential energy is defined for conservative forces. In contrast, when work is done by a non-conservative force, such as the frictional drag force opposing the sliding motion of an object down an inclined plane, that energy is dissipated, meaning it is not converted into a form which is available to do further mechanical work. The proper treatment of conservative and non-conservative becomes an essential approach in problem solving when incorporated with the broader principal of the conservation of energy. See also: Conservation of energy; Work
Units of force
Force has dimensions of mass (M) times length (L), divided by time (T) squared, or MLT-2. The Newton (N) is the force unit in the International System (SI) of units based on measurement of length in meters (m), time in seconds (s), and mass in kilograms (kg). A Newton is defined to be the force that will cause an object having a mass of 1 kilogram to have an acceleration of 1 m/s2. Other common units of force include pounds and dynes. See also: Units of measurement





