Sun as reference

The fundamental reference for the properties of stars is the Sun. The closest star to Earth, the Sun is located an average distance of 150 million km (93 million mi) away, a distance defined as the astronomical unit (AU). The Sun's diameter is approximately 1.4 million km (865,000 mi). From the energy received on Earth (1367 W m⁻²), the solar luminosity is 3.85 × 10²⁶ W. The surface temperature, where the “surface” is defined as where the solar gases become opaque, is 5777 K (9939°F). The Earth's orbital parameters and Kepler's third law give a solar mass of 1.99 × 10³⁰ kg, the solar volume leading to an average density of 1.4 g cm⁻³ (1.4 times that of water). See also: Astronomical unit; Heat radiation; Sun

The Sun's temperature and density rise inwardly as a result of gravitational compression, reach values at the solar center of 15.7 million K (28.3 million°F) and 151 g cm⁻³. Compositionally, the Sun is 91.5% hydrogen (by number of atoms), 8.5% helium, and about 0.015% everything else (oxygen dominating, then carbon, neon, and nitrogen), all in an ionized state of matter known as plasma. The solar chemical composition provides a standard for other stars as well as an observational benchmark against which to compare theories of the chemical evolution of the Milky Way Galaxy. See also: Milky Way Galaxy; Plasma (physics)

Above about 8 million K (14 million°F), within the inner quarter of the Sun's radius and about half the mass, hydrogen fuses to helium primarily via the proton-proton chain.

The resulting release of energy supports the Sun against gravitational contraction. The radiation works its way out, degrading in energy with decreasing temperature, until it is released largely at optical wavelengths. Nearly massless particles, called neutrinos, exit the Sun at close to the speed of light. Though nearly everything is transparent to them, enough are captured by terrestrial neutrino telescopes to confirm the theory of the Sun's energy production. There was initially enough hydrogen to run the chain for 10 billion years. With the Sun being 4.6 billion years old (from radioactive dating of meteorites), it has over 5 billion years of core hydrogen fusion left. See also: Neutrino; Positron; Proton-proton chain; Solar neutrino

Names

The brightest stars in the night sky carry proper names from ancient times, most of Arabic origin that reflect the position of the star within its classical or Arabic constellation. A more general system (from German celestial cartographer Johannes Bayer in the early seventeenth century) names stars within constellations by Greek letters roughly in accord with apparent brightness, followed by the Latin genitive of the constellation name (for example, Vega, in Lyra, is also Alpha Lyrae). More generally yet, brighter stars carry numbers, derived from the work of the English astronomer and writer John Flamsteed (1646–1719), in easterly order within a constellation (Vega also 3 Lyrae). All naked-eye stars also have HR (Harvard Revised) numbers assigned in order east of the vernal equinox. A variety of catalogs list millions of telescopic stars. See also: Astronomical catalogs; Constellation

Magnitudes and colors

About 130 BCE, Greek astronomer and mathematician Hipparchus assigned naked-eye stars to six brightness groups or “apparent magnitudes” (m), with first magnitude the brightest (Table 1). This scheme is now quantified as a logarithmic system such that five magnitudes correspond to a factor of 100 in brightness, rendering first magnitude 2.512… times brighter than second, and so on; calibration extends the brightest stars into negative numbers. See also: Magnitude (astronomy)

Stars assume subtle colors from red to blue-white, reflecting different spectral energy distributions that result from temperatures ranging from under 2000 K (3100°F) to over 100,000 K (180,000°F). The magnitude of a star therefore depends on the detector's color sensitivity. A blue-sensitive detector “sees” blue stars brighter than does the eye, which is most sensitive to yellow light, and will assign them lower magnitudes. Numerous magnitude systems range from the ultraviolet into the infrared, though the apparent visual magnitude (m_v = V) is still standard. The differences among the systems allow measures of stellar color and temperature. The total brightness at the Earth across the spectrum is given by the apparent bolometric magnitude (m_bol), which is dependent on the visual magnitude and temperature. See also: Color index

Distances

The distances and certain visual properties of the 10 closest known stars and brown dwarfs (described later in this article) are provided in Table 2. The fundamental means of finding stellar distances is parallax. As the Earth moves in orbit around the Sun, a nearby star will appear to shift its location against the background. Parallaxes of hundreds of thousands of stars were obtained with the European Space Agency's Hipparcos satellite, an astrometry mission that operated from 1989 to 1993, and allowed fair accuracy to distances of over 1000 light-years. Parallax measures then allow the calibration of other distance methods (such as spectroscopic distance, main-sequence cluster fitting, and use of Cepheid variables) that extend much farther. The successor to the Hipparcos mission, ESA's Gaia mission, launched in 2013. See also: Astrometry; Gaia mission; Hipparcos mission; Light-year; Parallax (astronomy); Parsec

Distribution and motions

All of stars visible with the unaided eye, along with some 300 billion more, are collected into the Milky Way Galaxy (or simply, the Galaxy). Approximately 98% of the Galaxy's stars are concentrated into a thin disk over 100,000 light-years across. From our perspective around Sun, located inside this disk and 27,000 light-years from the Galaxy's center in the constellation Sagittarius, the disk appears as the luminous, Milky Way band in the night sky. The appearance of the Milky Way varies considerably. It is brightest and widest toward the galactic center, and faintest in the opposite direction toward the anticenter in the constellations Taurus and Auriga (Fig. 2). Surrounding the disk is a vast halo that is sparsely populated by stars. See also: Constellation; Milky Way Galaxy

Fig. 2 Looking toward the core of Milky Way, along a line of sight holding millions of stars in the disk of the Galaxy and obscuring dark bands of dust. (Credit: NASA)

Because stars orbit the Galaxy's center, they are seen to move relative to the Sun. Statistical analysis of these motions shows the Sun to be moving through the local stars at a speed of 15–20 km/s (9–12 mi/s), roughly toward the star Vega. From radial velocities of sources outside the Galaxy, it is found that the Sun moves in a roughly circular orbit at 220 km/s (137 mi/s), which when combined with the space motions of other stars allows their galactic orbits to be determined. Stars in the disk have closely circular orbits; those in the extended spherical halo of the galaxy have elliptical ones. See also: Doppler effect

Absolute magnitudes

The apparent visual magnitude of a star depends on its intrinsic visual luminosity and on the inverse square of the distance. Knowledge of the distance allows the determination of the true visual luminosity, expressed as the absolute visual magnitude, M_v. This quantity is defined as the apparent visual magnitude that the star would have at a distance of 10 parsecs (abbreviated pc, equal to 3.26 light-years), and is found from the magnitude equation (1).

(1)

Absolute visual magnitudes from class O to mid L range from M_v = −10 to +23 (a factor of 10¹³). The Sun's absolute visual magnitude is in the middle of this range, +4.82. When the invisible infrared radiation from faint cool stars and the equally invisible ultraviolet from the ultraluminous hot stars are taken into account, the range in absolute bolometric luminosities (expressed in magnitudes as M_bol) is 30 times lower. The quantity M_bol can be calibrated in watts; for the Sun, M_bol = +4.74. See also: Magnitude (astronomy)

Spectral classes

Stars exhibit a variety of absorption-line spectra. The absorptions, narrow cuts in the spectra, are produced by atoms, ions, and molecules in the stars' thin, semitransparent outer layers, or atmospheres. Some stars show powerful hydrogen absorptions, others weak hydrogen and metals, yet others molecules but no hydrogen. Starting in the 19th century, U.S. astronomer Edward C. Pickering lettered them according to the strengths of their hydrogen lines. After he and his assistants dropped some letters and rearranged others for greater continuity, they arrived at the standard spectral sequence, OBAFGKM, which was later decimalized (the Sun is in class G2). Classes L and T were added in 1999, with class Y later adopted in the 2000s (Table 3). Since the sequence correlates with color, it must also correlate with temperature, which ranges from near 50,000 K (90,000°F) for hot class O, through about 6000 K (10,000°F) for solar-type class G, to under 2400 K (3860°F) for class L, and below 1400 K (2060°F) for class T (all of which are brown dwarf substars). See also: Color vision; Hydrogen; Ion; Molecule; Spectral type

The majority of stars in the galactic disk have chemical compositions like that of the Sun. Differences in spectra result primarily from changes in molecular and ionic composition and in the efficiencies of absorption, all of which correlate with temperature. Molecules exist only at low temperatures. At higher temperatures they break up, and neutral atoms become ionized, so the absorptions of the neutral state eventually weaken with increasing temperature. When the temperature becomes high enough, single ionization is replaced by double, and so on. Hydrogen reaches maximum strength in class A. Hotter than class A, hydrogen becomes ionized and the neutral hydrogen lines weaken in classes B and O. Even the absorptions of individual ions are affected, as absorbing electrons are pumped toward higher-energy states at higher temperatures. See also: Electron

Most stars have more oxygen than carbon. In some cool stars, however, the ratio is reversed, carbon molecules replacing those of metallic oxides. Stars whose temperatures track class M are assigned class N, warmer ones class R; both are now called carbon stars and lumped into class C. In between, where oxygen and carbon are in equal abundance, leftover oxygen attaches preferentially to zirconium (whose abundance is also raised above normal); the zirconium oxide stars are called class S. See also: Astronomical spectroscopy; Carbon star

Hertzsprung-Russell diagram

Shortly after the invention of the spectral sequence, scientists showed that luminosity correlates with spectral class. Between 1911 and 1913, Danish astronomer and physicist Ejnar Hertzsprung and U.S. astronomer Henry Norris Russell independently developed a graph of absolute visual (or bolometric) magnitude (luminosity increasing upward) plotted against spectral class (or temperature, decreasing toward the right), now known as the Hertzsprung-Russell (HR) diagram (Fig. 3). The majority of stars lie in a band in which luminosity climbs up and to the left with temperature (with the Sun in the middle). But another band begins near the location of the Sun and proceeds up and to the right (to lower temperature), luminosities increasing to thousands of times the mass of the Sun as temperature drops to class M. To be bright and cool, such stars must have large radiating areas and radii. To distinguish between the bands, the larger stars are called giants, while those of the main band are termed dwarfs (or main-sequence stars). Most carbon stars are giants, and stars of classes R, N, and S are mixed in with those of class M. Yet brighter stars to the cool side of the main sequence, with luminosities approaching a million solar, are called supergiants. In between the giants and the dwarfs lie a few subgiants. At the top, superior to the supergiants, are the very rare hypergiants. See also: Dwarf star; Giant star; Subgiant star; Supergiant star

Fig. 3 The Hertzsprung-Russell (HR) diagram, which plots stars' temperatures (x axis) against their luminosities (y axis). Numerous examples of stars in the Milky Way are included in this sample diagram. (Credit: ESO)

Stellar sizes

Since, for any spherical star, luminosity L = 4π R²σ T⁴, the luminosity in solar units is given by Eq. (2).

(2)

Temperature (T) from spectral class or spectral analysis plus the luminosity from absolute bolometric magnitude M_bol gives the stellar radius. Dwarf radii increase from about 10% that of the Sun in class M to nearly 15 solar in class O. Giant radii range past 10 times solar to well over 1 AU, supergiants to 8 AU. White dwarfs are about the size of Earth. The radii of stars are confirmed by direct measurement of angular size through interferometry and analysis of eclipsing double (binary) stars. See also: Eclipsing variable stars

Spectral lines are sensitive to stellar density, so stars can be classified by size and luminosity on the basis of their spectra alone. In the standard Morgan-Keenan-Kellman (MKK) system, roman numerals I through V stand for supergiant, bright giant, giant, subgiant, and dwarf (VI is sometimes used for subdwarf). Once luminosity and absolute visual magnitude are known from a star's spectrum, its spectroscopic distance can be calculated from the magnitude equation (which must contain a correction for dimming by interstellar dust).

Double and multiple stars

Most stars are members of some sort of community, from doubles through multiples (double-doubles, and so forth) to clusters, which themselves contain doubles. Separations between components of double stars range from thousands of AUs (with orbital periods of a million years) to stars that touch each other (and orbit in hours). Orbits of the more widely spaced binaries (periods of months to centuries) can be determined directly (or through interferometry). Limited orbital parameters of the more closely spaced ones are found by means of Doppler shifts caused by high orbital velocities. Some of the latter eclipse each other, the eclipses establishing orbital tilts, true orbital speeds, and stellar radii. The sum of the masses of a binary is found from the period and orbital dimension through Kepler's third law; the ratio of the masses is found by locating the center of mass of the system; and the combination of these two leads to determination of the individual masses. See also: Binary star; Kepler's laws; Orbital motion

Masses and main-sequence properties

Observations of hundreds of binary stars show that mass (M) increases upward along the main sequence from about 7.5% solar mass at cool class M to over 20 solar masses in the cooler end of class O. Extrapolation by theory, as well as through observations of clusters, suggests solar masses of 200 or more at the extreme hot end (class O2–O3) of the main sequence. Fainter than the Sun, luminosity is proportional to M³; from the Sun and brighter, luminosity goes as M⁴ and then back to M³. This mass-luminosity relation is the result of higher internal temperatures and pressures in more massive stars caused by gravitational compression. Given the Sun's energy source and that luminosity varies smoothly with mass, the main sequence must be a hydrogen-fusing mass sequence. Giants, supergiants, and white dwarfs must therefore have some other energy source. See also: Mass-luminosity relation

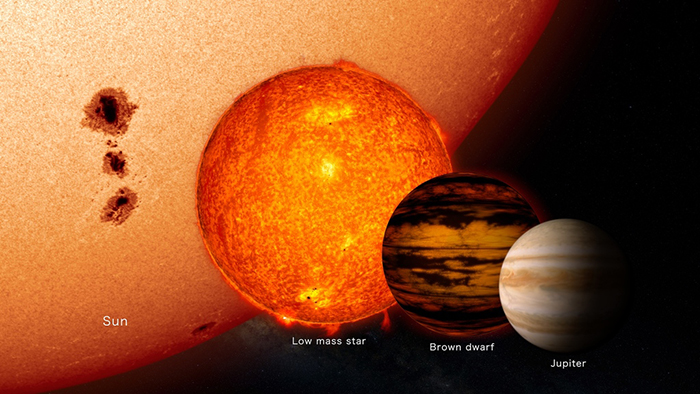
Brown dwarfs

Below a lower mass limit of 7.5% solar, internal temperatures and densities are not great enough to allow the proton-proton chain to operate. Such stars, called brown dwarfs, glow dimly and redly from gravitational contraction and from the fusion of natural deuterium (²H) into helium (Fig. 4). Brown dwarfs and the stars at the bottom of the main sequence together inhabit class L. All class T stars are brown dwarfs. The lower mass limit to brown dwarfs is unknown; they may even overlap the masses of planets. See also: Brown dwarf; Exoplanet; Infrared astronomy

Fig. 4 An artist's impression of the sizes and appearances of a typical Sunlike star, a low-mass star, a brown dwarf, and a gas giant planet. (Credit: NASA’s Goddard Space Flight Center)

Clusters

Stars are often grouped together in clusters, with the stellar member orbiting a common center of mass. The two basic kinds of clusters are open clusters and globular clusters. Open clusters are fairly small collections in which a few hundred or a thousand stars are scattered across a few tens of light-years. Examples are the Pleiades and Hyades in Taurus, which are among thousands of such clusters occupying the Galaxy's disk (and thus the Milky Way) (Fig. 5).

Fig. 5 The Jewel Box open cluster (Kappa Crucis), which lies next to the Crux (Southern Cross) constellation. (Credit: ESO)

Globular clusters are generally spherical, dense collections of stars, numbering as high as a few million within a volume just 100 light-years or so across (Fig. 6). About 150 globular clusters occupy the Galaxy's halo. The main-sequence stars of globular clusters are subdwarfs, and like other halo stars are deficient in metals. See also: Globular clusters

Fig. 6 Globular cluster 47 Tucanae, one of the grandest in the Milky Way Galaxy, containing over 1 million stars, seen here by the Hubble Space Telescope. [Credit: NASA, ESA, and the Hubble Heritage (STScI/AURA)-ESA/Hubble Collaboration. Acknowledgment: J. Mack (STScI) and G. Piotto (University of Padova, Italy)]

The HR diagrams of clusters are radically different from the HR diagrams of the general stellar field. Those of open clusters differ among themselves in having various portions of the upper main sequence removed. Some main sequences go all the way from class M to O, while others extend only from M through G. The effect is related to the cluster's age, since high-mass stars die first. Globular clusters, which lack an upper main sequence and are therefore all old (up to about 12.5 billion or so years), contain a distinctive “horizontal branch” composed of modest giants. See also: Star clusters

Variable stars

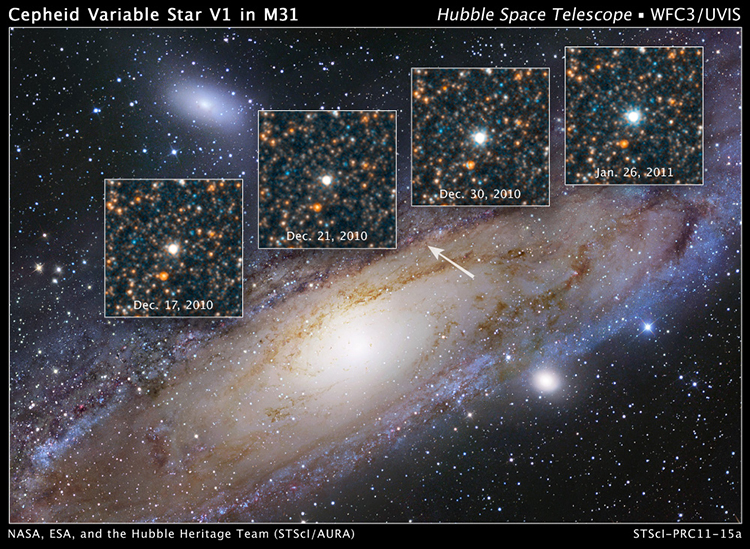
Dwarfs are generally stable. Giants and supergiants, however, can have structures that allow them to pulsate. Cepheids (named after the star Delta Cephei) are F and G supergiants and bright giants that occupy a somewhat vertical instability strip in the middle of the HR diagram. They vary regularly by about a magnitude over periods ranging from 1 to 50 days. The pulsation is driven by a deep layer of gas in which helium is becoming ionized. This layer alternately traps and releases heat from the outgoing radiation. Larger and more luminous Cepheids take longer to pulsate. Once this period-luminosity relationship is calibrated through parallax and main-sequence cluster fitting, the period of a Cepheid gives its absolute magnitude, which in turn gives its distance. Cepheids are vital in finding distances to other galaxies (Fig. 7). See also: Cepheid variable star; Hubble constant

Fig. 7 Hubble Space Telescope images of the star known as V1, which U.S. astronomer Edwin Hubble (the telescope's namesake) discovered in 1923. Hubble used this Cepheid variable to show that a faint, fuzzy patch in the constellation Andromeda is actually another galaxy outside of our own, the Milky Way. The space telescope images show the star's changing brightness over a short time period. [Credit: NASA, ESA, and the Hubble Heritage Team (STScI/AURA)]

As the instability strip crosses the class F and A giants found in globular clusters, it produces the low-metal RR Lyrae stars, which have periods of a day or less. Since they all have absolute magnitudes around 0.7, they too are valuable distance indicators. Farther down the HR diagram, the instability strip crosses the class A and F main sequence. Here and in the giants just above it are the subtly varying Delta Scuti stars. Where the instability strip hits the hydrogen-rich white dwarfs, at temperatures between 10,500 and 13,000 K (18,400 and 22,900°F), it produces the ZZ Ceti stars, which pulsate nonradially with periods of minutes. (Hydrogen-poor white dwarfs have their own instability zone around 20,000 K or 35,540°F.)

Miras (after the star Mira, Omicron Ceti), or long-period variables, are luminous M, C, or S giants that can vary visually by more than 10 magnitudes over periods that range from 50 to 1000 days, the pulsations again driven by deep ionization layers. Much of the visual variation is caused by small changes in temperature that shift the starlight from the visual to the infrared and that also alter the formation of obscuring titanium oxide molecules. The infrared (and bolometric) variation amounts to only two magnitudes or so. Other giants, and especially supergiants, are semiregular or even irregular variables with no periodicities at all. Still other stars, such as the class B subgiant Beta Cephei stars, are multi-periodic. See also: Mira

Duplicity produces its own set of intrinsic variations. If the members of a binary system are close enough together, one of them can transfer mass to the other, and instabilities in the transfer process cause the binary to flicker. If one companion is a white dwarf, infalling compressed hydrogen can erupt in a thermonuclear runaway, producing a sudden nova that can reach absolute magnitude −10. If the white dwarf gains enough matter such that it exceeds its allowed limit (the Chandrasekhar limit) of 1.4 solar masses, it can even explode as a supernova (discussed below). See also: Cataclysmic variable; Nova; Variable star

Off the HR diagram

Various kinds of stars are not placeable on the classical HR diagram. The most common examples are the central stars of planetary nebulae, which are complex shells and rings of ionized gas that surround hot blue stars that range in temperature from 25,000 to over 200,000 K (45,000 to over 360,000°F) (Fig. 8). While they can have luminosities more than 10,000 solar, these central stars appear relatively dim to the eye, as they produce most of their radiation in the high-energy ultraviolet portion of the electromagnetic spectrum. This radiation ionizes the surrounding nebulae and causes them to glow. On the theoretician's extended HR diagram, in which luminosity is plotted directly against temperature, the planetary nebula central stars clearly connect the red giants with the white dwarfs. See also: Planetary nebula

Fig. 8 Hubble Space Telescope image of the Ring Nebula in Lyra, a planetary nebula made of glowing ionized gas that surrounds a hot blue star. [Credit: H. Bond et al., Hubble Heritage Team (STScI /AURA), NASA]

Even hotter neutron stars are found associated with the exploded remains of supernovae (supernova remnants). Only 25 or so kilometers (15 mi) across, the visible ones spin rapidly, and are highly magnetic, with fields a trillion times the strength of Earth's field. Radiation beamed from tilted, wobbling magnetic axes can strike the Earth to produce seeming pulses of radiation (Fig. 9). Over time, the spin rates slow and these so-called pulsars disappear. The remains of exploded stars, quiet pulsars (and active ones whose radiation does not hit Earth) litter the Galaxy. Rare magnetars can have fields 100 times those of normal neutron stars. See also: Crab Nebula; Neutron star; Pulsar

Fig. 9 A multi-wavelength view of the Crab Nebula. The pulsar at its heart appears as a white dot in this image. The image compiles observations in radio waves via the Karl G. Jansky Very Large Array (VLA), infrared light via the Spitzer Space Telescope. Visble light via the Hubble Space Telescope, and x-rays via the Chandra X-ray Observatory. [Credit: NASA, ESA, G. Dubner (IAFE, CONICET-University of Buenos Aires) et al.; A. Loll et al.; T. Temim et al.; F. Seward et al.; VLA/NRAO/AUI/NSF; Chandra/CXC; Spitzer/JPL-Caltech; XMM-Newton/ESA; and Hubble/STScI]

Evolution

The different kinds of stars can be linked through theories of stellar evolution. Stars are born by the gravitational collapse of dense knots within cold dusty molecular clouds found only in the Galaxy's disk. When the new stars are hot enough inside to initiate fusion, their contraction halts and they settle onto the main sequence. The higher the stellar mass, the greater the internal compression and temperature, and the more luminous the star. But the higher the internal temperature, the greater the rate at which hydrogen is fused, and the shorter the star's life. The fuel supply is proportional to mass, M, and the rate of fusion is proportional to the luminosity, L. Lifetime t is proportional to (fuel supply)/(rate of use) and thus to M/L. But L is on average proportional to M^3.5, so t is proportional to 1/M^2.5. For example, a 100 solar mass star will exhaust its core hydrogen in only 2.5 million years. See also: Molecular cloud; Protostar

Main-sequence lifetime is so long for stars under 0.8 to 0.9 solar mass that none has ever evolved in the history of the Galaxy. When a star between 0.8 and about 9 solar masses uses all its core hydrogen, its outer layers expand and cool to class K. The star then brightens as it becomes a giant, lower masses brightening by larger factors after first becoming subgiants. The ascent to gianthood is terminated when the central temperature is high enough (100 million K or 180 million°F) to initiate the fusion of core helium via the triple-alpha process, wherein three helium nuclei (alpha particles) combine to make one carbon atom. This process temporarily stabilizes the star with a helium-burning core and a hydrogen-burning shell. Fusion with another ⁴He nucleus makes oxygen. See also: Alpha particles; Helium

When the helium is fused to carbon and oxygen, the core contracts, helium fusion spreads outward into a shell, and the star again climbs the giant branch, the hydrogen- and helium-fusing shells sequentially turning on and off. Such asymptotic giant branch (AGB) stars become larger and brighter than before, passing into class M where they eventually become unstable enough to pulsate as Miras. Within a certain mass range, convection in giants can bring carbon from helium fusion (as well as other new elements) to the surface, and the star can become a class S and then a carbon star. Powerful winds strip the star nearly to its fusion zone, which is protected from the outside by a low-mass hydrogen envelope. As the inner region becomes exposed, the star heats and eventually illuminates the surrounding outflowing matter to produce a planetary nebula, which dissipates into the interstellar medium, leaving a carbon-oxygen white dwarf behind.

When high-mass stars, those above about 8 to 10 solar masses, use up their core hydrogen, they too migrate to the right on the HR diagram, becoming not so much brighter but larger, cooling at their surfaces and turning into supergiants. Below about 40 solar masses they become class M red supergiants, losing huge amounts of matter through immense winds. Some stabilize there under the action of helium fusion; others loop back to become blue supergiants. Above 60 solar masses, so much mass is lost that the supergiants do not make it much past class B, stalling there as helium fusion begins. Supergiants are so massive that, whatever their outward disposition, when the internal helium is fused to carbon and oxygen, the core shrinks and heats to the point that carbon fusion can begin. The mixture converts to oxygen, neon, and magnesium, which then burns to a mixture of silicon and sulfur, and finally to iron, each fusion stage moving outward to surround the core in successive shells (Fig. 10).

Fig. 10 An artist's impression of a cutaway into an aged, high-mass star, offering a simplified depiction of its internal elemental layering. Elements are identified by their periodic table symbol. (Credit: NASA/CXC/M.Weiss)

Because iron cannot fuse and produce energy, the core suddenly collapses and somehow “bounces” to explode away all the outer layers. From the outside, the observer sees the explosion as a core collapse supernova that can reach absolute magnitude −18. Temperatures within the exploding layers are high enough to create all the chemical elements, including iron, nickel, and uranium. The debris—the supernova remnant—expands for centuries into space, its shock wave helping to compress the interstellar matter and create new stars. The remaining core is so dense that it converts to a neutron star or, if the mass is great enough, even to a black hole. See also: Black hole; Crab Nebula; Nebula

Other routes to stellar explosion derive from double stars. Mass transfer from a low-mass main-sequence star to a white dwarf companion can cause the white dwarf to exceed the Chandrasekhar limit of 1.4 solar masses (beyond which it cannot support itself by way of electron degeneracy). The resultant collapse of the white dwarf produces a hydrogenless type Ia supernova that can reach M_v = −19 with such reliability that such supernovae are used to measure distances to distant galaxies. Stellar evolution feeds huge quantities of enriched matter back into the star-generating clouds of interstellar space, causing the metal content of the Galaxy to increase and providing the raw materials from which the Earth is made. See also: Earth; Nucleosynthesis; Stellar evolution; Supernova

James B. Kaler